878 APPENDIX C. LAGRANGIAN MECHANICS

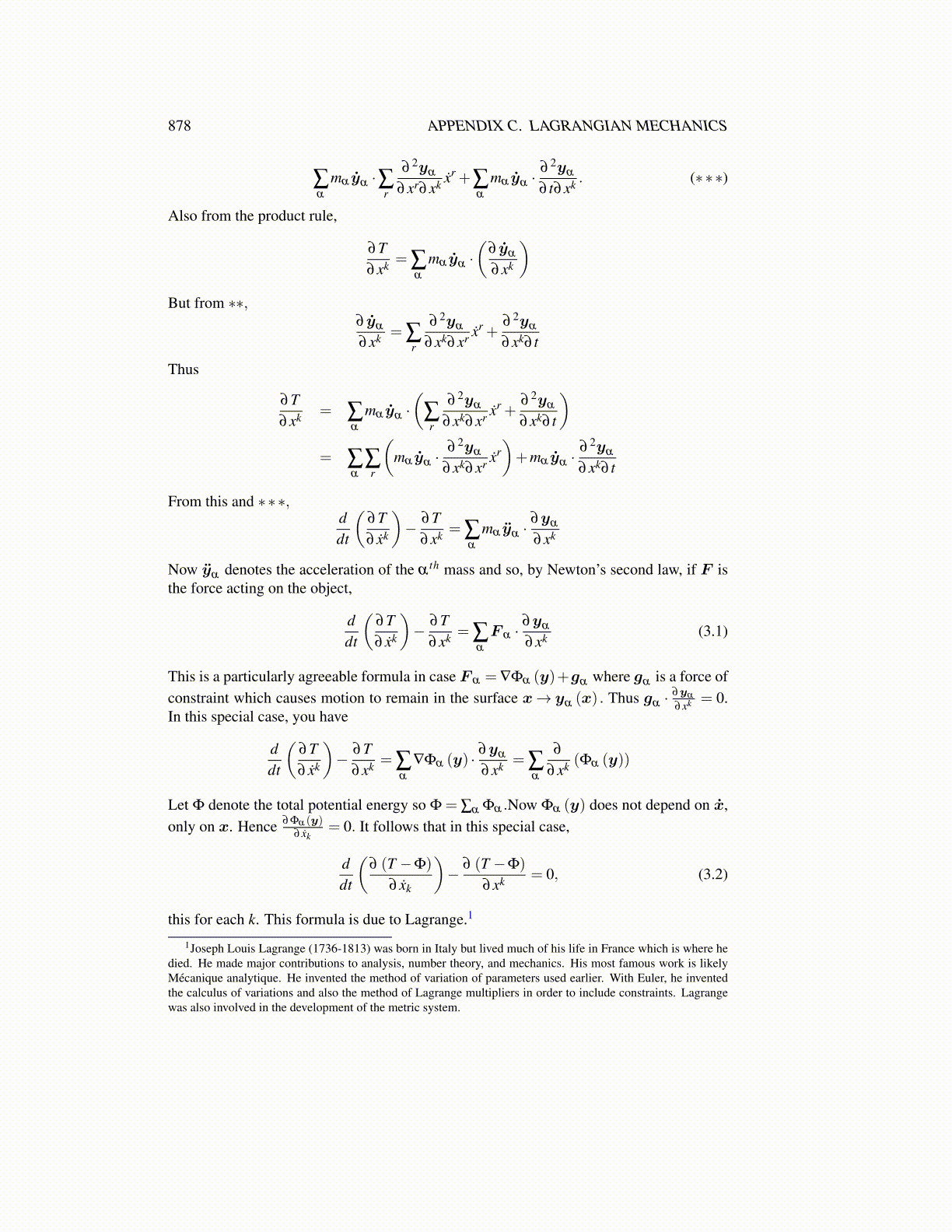
∑α

mα ẏα ·∑r

∂ 2yα

∂xr∂xk ẋr +∑α

mα ẏα ·∂ 2yα

∂ t∂xk . (∗∗∗)

Also from the product rule,

∂T∂xk = ∑

α

mα ẏα ·(

∂ ẏα

∂xk

)But from ∗∗,

∂ ẏα

∂xk = ∑r

∂ 2yα

∂xk∂xr ẋr +∂ 2yα

∂xk∂ t

Thus

∂T∂xk = ∑

α

mα ẏα ·(

∑r

∂ 2yα

∂xk∂xr ẋr +∂ 2yα

∂xk∂ t

)= ∑

α

∑r

(mα ẏα ·

∂ 2yα

∂xk∂xr ẋr)+mα ẏα ·

∂ 2yα

∂xk∂ t

From this and ∗∗∗,ddt

(∂T∂ ẋk

)− ∂T

∂xk = ∑α

mα ÿα ·∂yα

∂xk

Now ÿα denotes the acceleration of the α th mass and so, by Newton’s second law, if F isthe force acting on the object,

ddt

(∂T∂ ẋk

)− ∂T

∂xk = ∑α

F α ·∂yα

∂xk (3.1)

This is a particularly agreeable formula in case F α = ∇Φα (y)+gα where gα is a force ofconstraint which causes motion to remain in the surface x→ yα (x) . Thus gα ·

∂yα

∂xk = 0.In this special case, you have

ddt

(∂T∂ ẋk

)− ∂T

∂xk = ∑α

∇Φα (y) · ∂yα

∂xk = ∑α

∂

∂xk (Φα (y))

Let Φ denote the total potential energy so Φ = ∑α Φα .Now Φα (y) does not depend on ẋ,only on x. Hence ∂Φα (y)

∂ ẋk= 0. It follows that in this special case,

ddt

(∂ (T −Φ)

∂ ẋk

)− ∂ (T −Φ)

∂xk = 0, (3.2)

this for each k. This formula is due to Lagrange.1

1Joseph Louis Lagrange (1736-1813) was born in Italy but lived much of his life in France which is where hedied. He made major contributions to analysis, number theory, and mechanics. His most famous work is likelyMécanique analytique. He invented the method of variation of parameters used earlier. With Euler, he inventedthe calculus of variations and also the method of Lagrange multipliers in order to include constraints. Lagrangewas also involved in the development of the metric system.