Appendix C

Lagrangian Mechanics

Let y = y (x,t) where t signifies time and x ∈U ⊆ Rm for U an open set, while y ∈ Rn

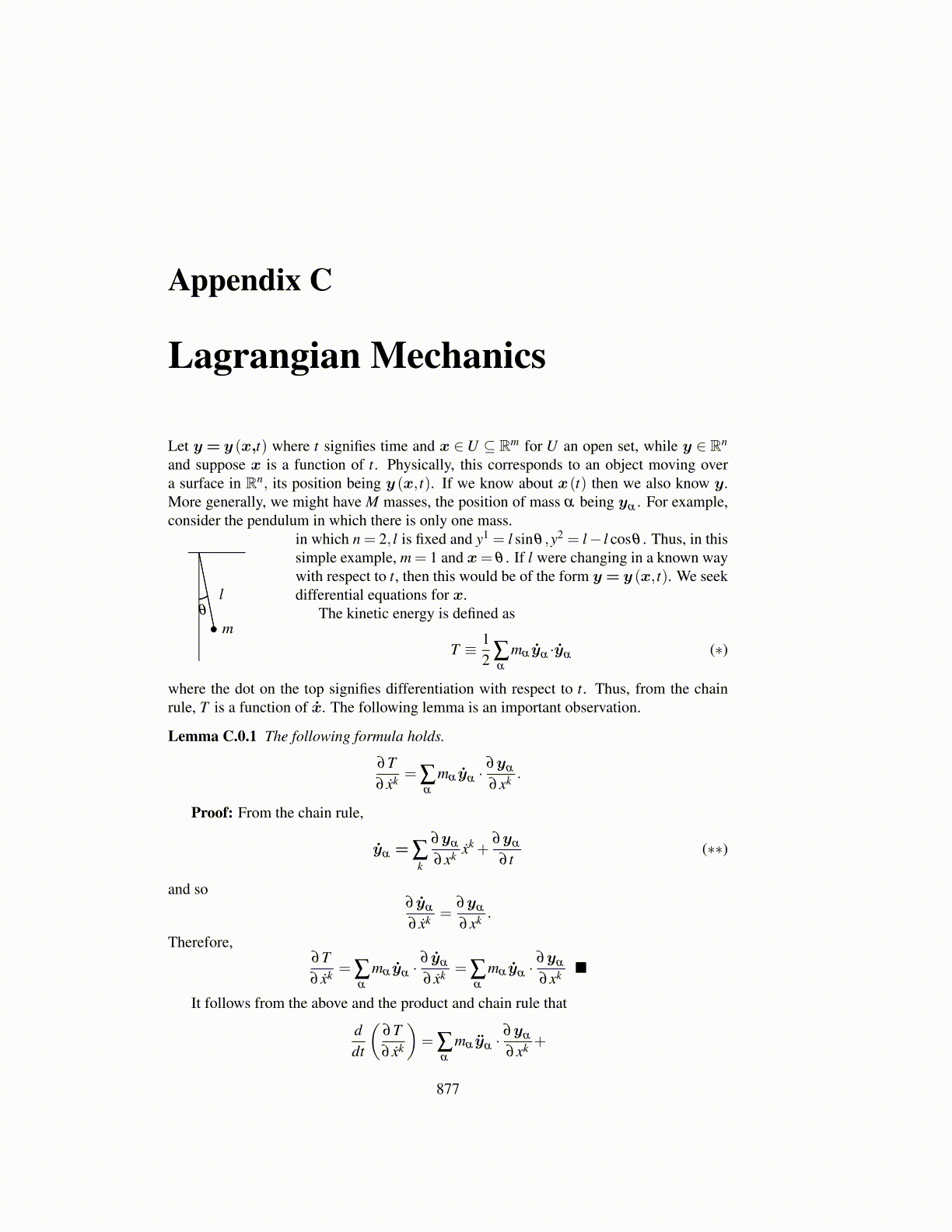
and suppose x is a function of t. Physically, this corresponds to an object moving overa surface in Rn, its position being y (x, t). If we know about x(t) then we also know y.More generally, we might have M masses, the position of mass α being yα . For example,consider the pendulum in which there is only one mass.

• m

lθ

in which n = 2, l is fixed and y1 = l sinθ ,y2 = l− l cosθ . Thus, in thissimple example, m = 1 and x= θ . If l were changing in a known waywith respect to t, then this would be of the form y = y (x, t). We seekdifferential equations for x.

The kinetic energy is defined as

T ≡ 12 ∑

α

mα ẏα ·ẏα (∗)

where the dot on the top signifies differentiation with respect to t. Thus, from the chainrule, T is a function of ẋ. The following lemma is an important observation.

Lemma C.0.1 The following formula holds.

∂T∂ ẋk = ∑

α

mα ẏα ·∂yα

∂xk .

Proof: From the chain rule,

ẏα = ∑k

∂yα

∂xk ẋk +∂yα

∂ t(∗∗)

and so∂ ẏα

∂ ẋk =∂yα

∂xk .

Therefore,∂T∂ ẋk = ∑

α

mα ẏα ·∂ ẏα

∂ ẋk = ∑α

mα ẏα ·∂yα

∂xk ■

It follows from the above and the product and chain rule that

ddt

(∂T∂ ẋk

)= ∑

α

mα ÿα ·∂yα

∂xk +

877