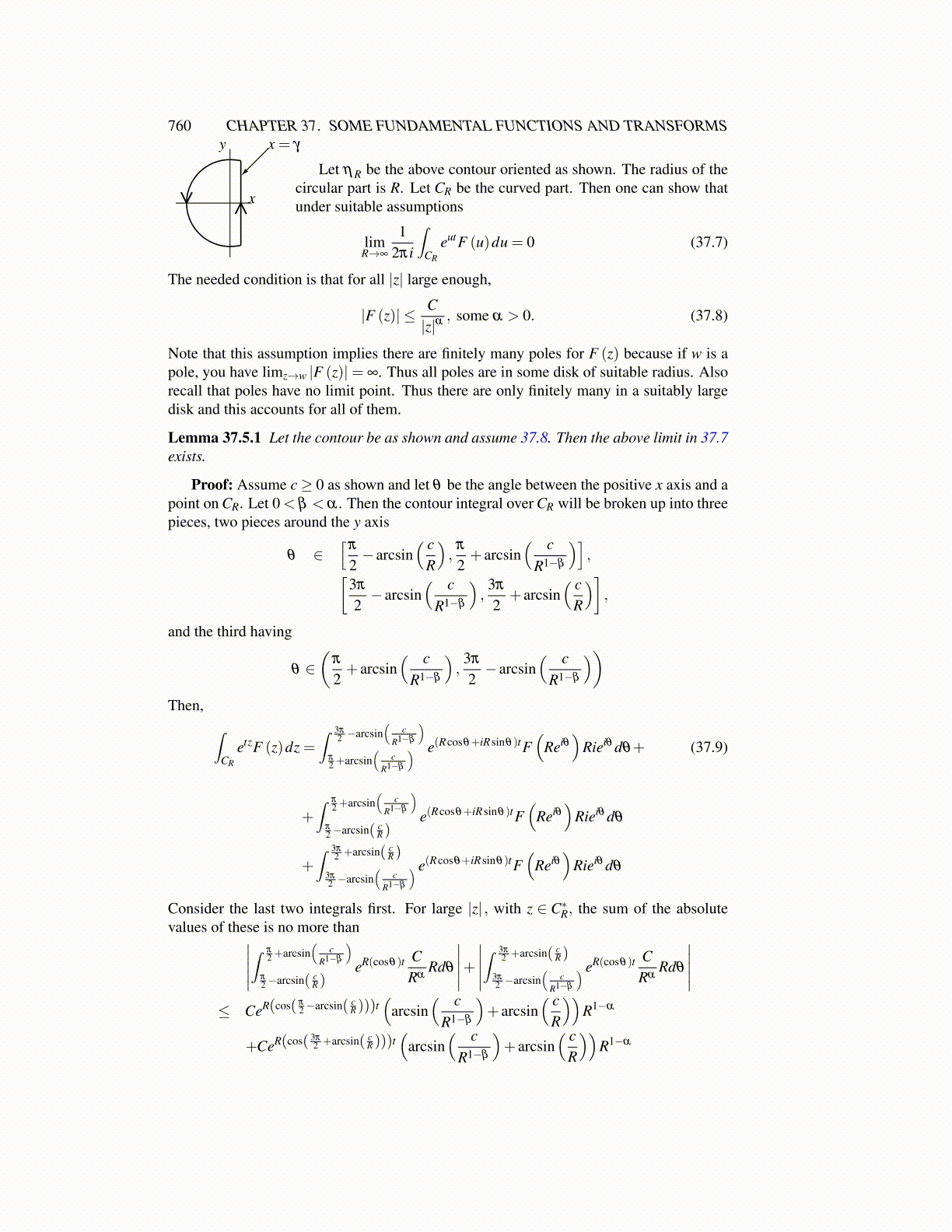
760 CHAPTER 37. SOME FUNDAMENTAL FUNCTIONS AND TRANSFORMS
x
x = γy
Let ηR be the above contour oriented as shown. The radius of thecircular part is R. Let CR be the curved part. Then one can show thatunder suitable assumptions
limR→∞
12πi
∫CR
eutF (u)du = 0 (37.7)
The needed condition is that for all |z| large enough,
|F (z)| ≤ C|z|α
, some α > 0. (37.8)
Note that this assumption implies there are finitely many poles for F (z) because if w is apole, you have limz→w |F (z)|= ∞. Thus all poles are in some disk of suitable radius. Alsorecall that poles have no limit point. Thus there are only finitely many in a suitably largedisk and this accounts for all of them.
Lemma 37.5.1 Let the contour be as shown and assume 37.8. Then the above limit in 37.7exists.
Proof: Assume c≥ 0 as shown and let θ be the angle between the positive x axis and apoint on CR. Let 0 < β < α . Then the contour integral over CR will be broken up into threepieces, two pieces around the y axis
θ ∈[
π
2− arcsin
( cR
),
π
2+ arcsin
( cR1−β
)],[
3π
2− arcsin
( cR1−β
),
3π
2+ arcsin
( cR
)],
and the third having
θ ∈(
π
2+ arcsin
( cR1−β
),
3π
2− arcsin
( cR1−β
))Then, ∫
CR
etzF (z)dz =∫ 3π
2 −arcsin(
cR1−β
)π2 +arcsin
(c
R1−β
) e(Rcosθ+iRsinθ)tF(
Reiθ)
Rieiθ dθ+ (37.9)
+∫ π
2 +arcsin(
cR1−β
)π2−arcsin( c
R )e(Rcosθ+iRsinθ)tF
(Reiθ
)Rieiθ dθ
+∫ 3π
2 +arcsin( cR )
3π2 −arcsin
(c
R1−β
) e(Rcosθ+iRsinθ)tF(
Reiθ)
Rieiθ dθ
Consider the last two integrals first. For large |z| , with z ∈ C∗R, the sum of the absolutevalues of these is no more than∣∣∣∣∣
∫ π2 +arcsin
(c
R1−β
)π2−arcsin( c
R )eR(cosθ)t C
RαRdθ
∣∣∣∣∣+∣∣∣∣∣∫ 3π
2 +arcsin( cR )
3π2 −arcsin
(c
R1−β
) eR(cosθ)t CRα
Rdθ
∣∣∣∣∣≤ CeR(cos( π
2−arcsin( cR )))t
(arcsin
( cR1−β
)+ arcsin
( cR
))R1−α
+CeR(cos( 3π2 +arcsin( c
R )))t(
arcsin( c
R1−β
)+ arcsin
( cR
))R1−α