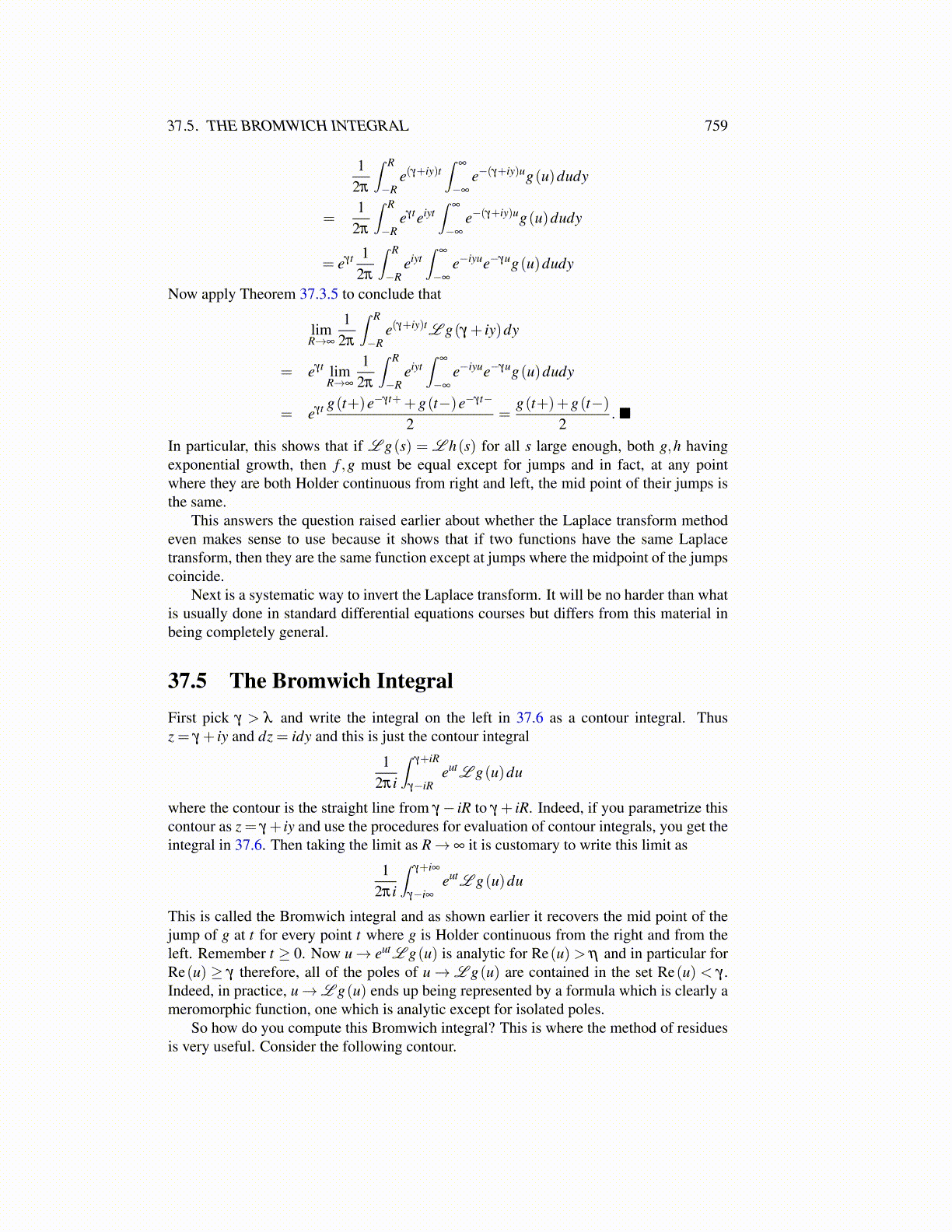
37.5. THE BROMWICH INTEGRAL 759
12π
∫ R
−Re(γ+iy)t
∫∞
−∞
e−(γ+iy)ug(u)dudy
=1
2π
∫ R
−Reγteiyt
∫∞
−∞
e−(γ+iy)ug(u)dudy
= eγt 12π
∫ R
−Reiyt∫
∞
−∞
e−iyue−γug(u)dudy
Now apply Theorem 37.3.5 to conclude that
limR→∞
12π
∫ R
−Re(γ+iy)tL g(γ + iy)dy
= eγt limR→∞
12π
∫ R
−Reiyt∫
∞
−∞
e−iyue−γug(u)dudy
= eγt g(t+)e−γt++g(t−)e−γt−
2=
g(t+)+g(t−)2
.■
In particular, this shows that if L g(s) = L h(s) for all s large enough, both g,h havingexponential growth, then f ,g must be equal except for jumps and in fact, at any pointwhere they are both Holder continuous from right and left, the mid point of their jumps isthe same.
This answers the question raised earlier about whether the Laplace transform methodeven makes sense to use because it shows that if two functions have the same Laplacetransform, then they are the same function except at jumps where the midpoint of the jumpscoincide.
Next is a systematic way to invert the Laplace transform. It will be no harder than whatis usually done in standard differential equations courses but differs from this material inbeing completely general.
37.5 The Bromwich IntegralFirst pick γ > λ and write the integral on the left in 37.6 as a contour integral. Thusz = γ + iy and dz = idy and this is just the contour integral
12πi
∫γ+iR
γ−iReutL g(u)du
where the contour is the straight line from γ− iR to γ + iR. Indeed, if you parametrize thiscontour as z = γ + iy and use the procedures for evaluation of contour integrals, you get theintegral in 37.6. Then taking the limit as R→ ∞ it is customary to write this limit as
12πi
∫γ+i∞
γ−i∞eutL g(u)du
This is called the Bromwich integral and as shown earlier it recovers the mid point of thejump of g at t for every point t where g is Holder continuous from the right and from theleft. Remember t ≥ 0. Now u→ eutL g(u) is analytic for Re(u)> η and in particular forRe(u) ≥ γ therefore, all of the poles of u→ L g(u) are contained in the set Re(u) < γ .Indeed, in practice, u→L g(u) ends up being represented by a formula which is clearly ameromorphic function, one which is analytic except for isolated poles.
So how do you compute this Bromwich integral? This is where the method of residuesis very useful. Consider the following contour.