A.4. WHICH FUNCTIONS ARE INTEGRABLE? 853

MQ j(g)mQ j(g)

Q j

xn+1

x

xn+1 = g(x) xn+1 = f (x)

0 0 0 0 0 0 0 0 0

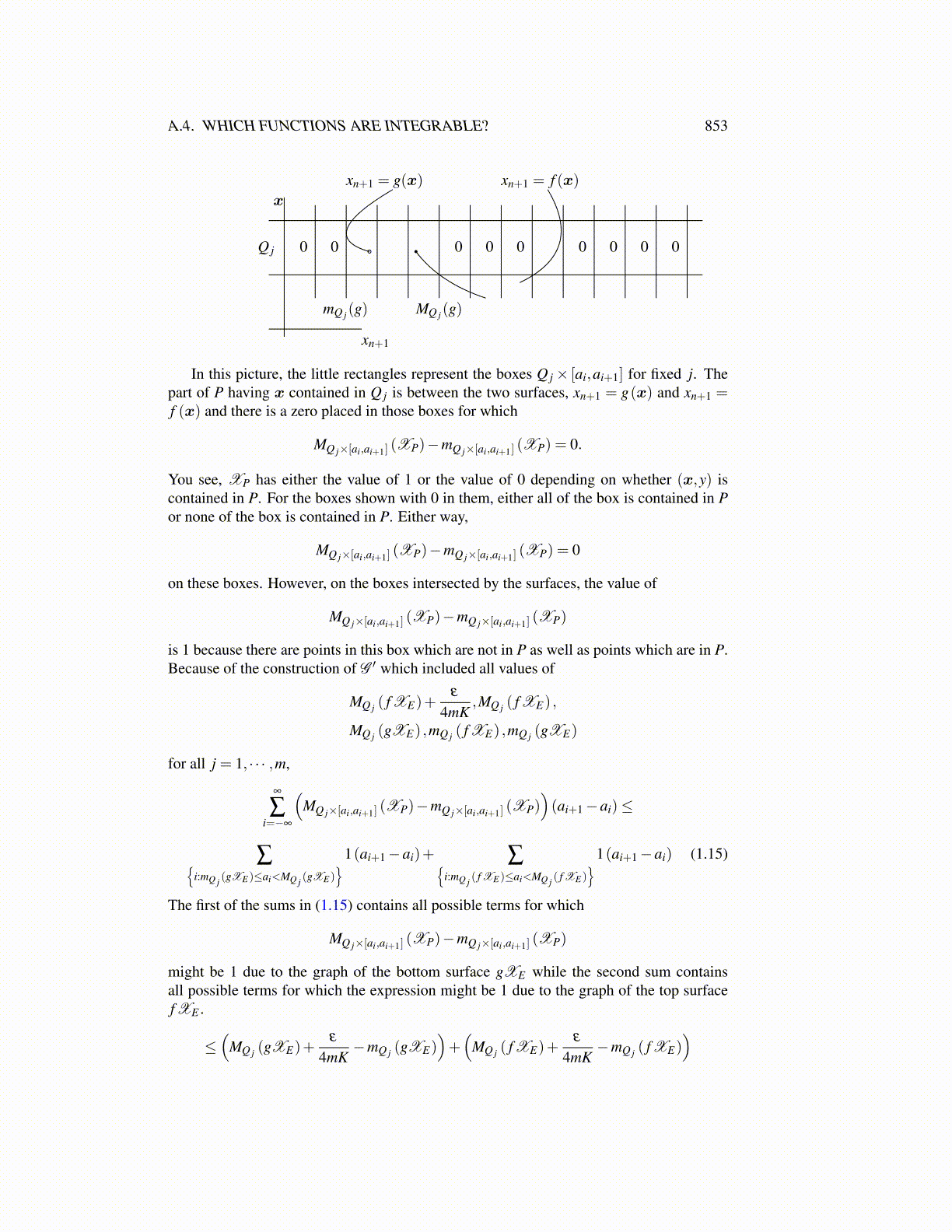
In this picture, the little rectangles represent the boxes Q j× [ai,ai+1] for fixed j. Thepart of P having x contained in Q j is between the two surfaces, xn+1 = g(x) and xn+1 =f (x) and there is a zero placed in those boxes for which

MQ j×[ai,ai+1] (XP)−mQ j×[ai,ai+1] (XP) = 0.

You see, XP has either the value of 1 or the value of 0 depending on whether (x,y) iscontained in P. For the boxes shown with 0 in them, either all of the box is contained in Por none of the box is contained in P. Either way,

MQ j×[ai,ai+1] (XP)−mQ j×[ai,ai+1] (XP) = 0

on these boxes. However, on the boxes intersected by the surfaces, the value of

MQ j×[ai,ai+1] (XP)−mQ j×[ai,ai+1] (XP)

is 1 because there are points in this box which are not in P as well as points which are in P.Because of the construction of G ′ which included all values of

MQ j ( f XE)+ε

4mK,MQ j ( f XE) ,

MQ j (gXE) ,mQ j ( f XE) ,mQ j (gXE)

for all j = 1, · · · ,m,

∞

∑i=−∞

(MQ j×[ai,ai+1] (XP)−mQ j×[ai,ai+1] (XP)

)(ai+1−ai)≤

∑{i:mQ j (gXE )≤ai<MQ j (gXE )

}1(ai+1−ai)+ ∑{i:mQ j ( f XE )≤ai<MQ j ( f XE )

}1(ai+1−ai) (1.15)

The first of the sums in (1.15) contains all possible terms for which

MQ j×[ai,ai+1] (XP)−mQ j×[ai,ai+1] (XP)

might be 1 due to the graph of the bottom surface gXE while the second sum containsall possible terms for which the expression might be 1 due to the graph of the top surfacef XE .

≤(

MQ j (gXE)+ε

4mK−mQ j (gXE)

)+(

MQ j ( f XE)+ε

4mK−mQ j ( f XE)

)