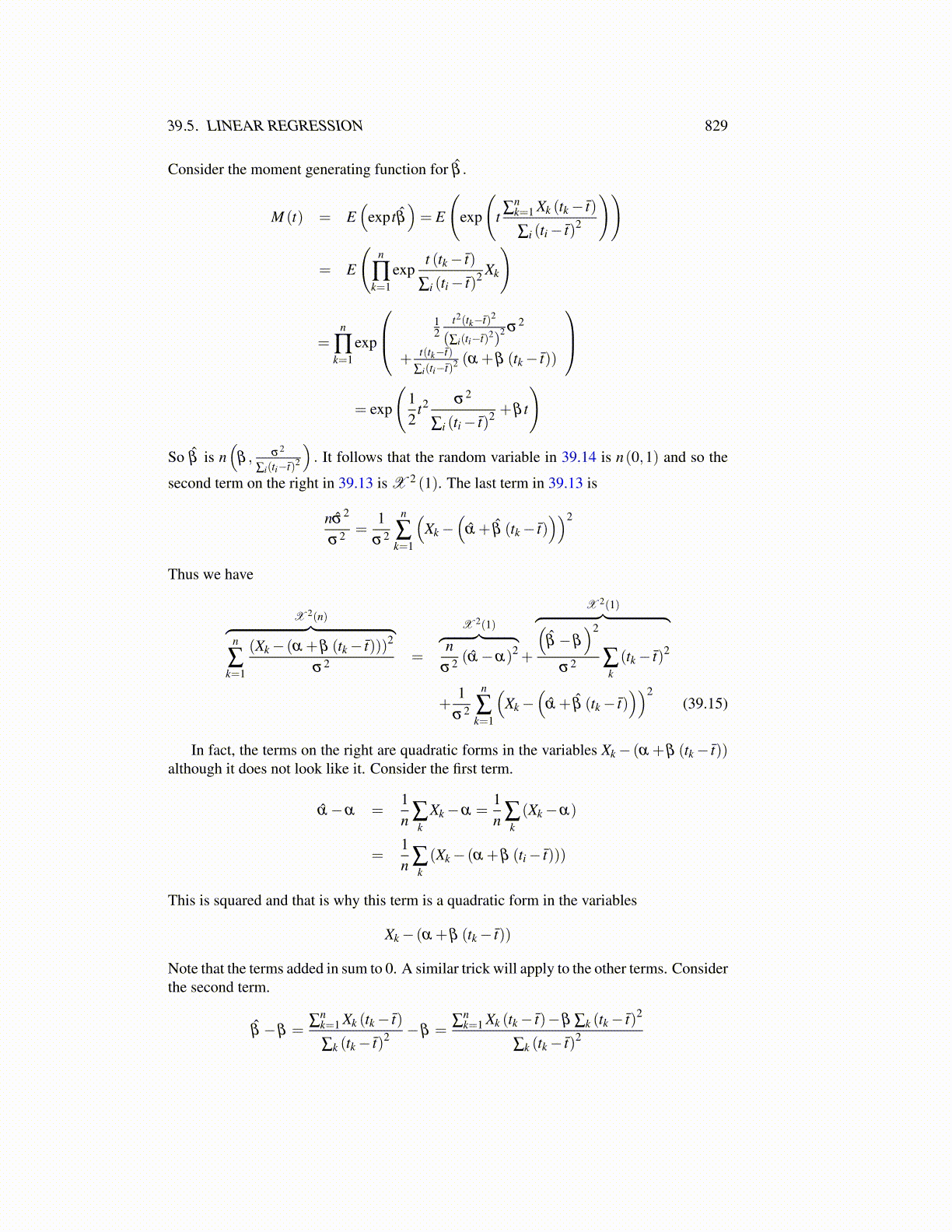
39.5. LINEAR REGRESSION 829

Consider the moment generating function for β̂ .

M (t) = E(

exp tβ̂)= E

(exp

(t∑

nk=1 Xk (tk− t̄)

∑i (ti− t̄)2

))

= E

(n

∏k=1

expt (tk− t̄)

∑i (ti− t̄)2 Xk

)

=n

∏k=1

exp

 12

t2(tk−t̄)2

(∑i(ti−t̄)2)2 σ2

+ t(tk−t̄)∑i(ti−t̄)2 (α +β (tk− t̄))

= exp

(12

t2 σ2

∑i (ti− t̄)2 +β t

)

So β̂ is n(

β , σ2

∑i(ti−t̄)2

). It follows that the random variable in 39.14 is n(0,1) and so the

second term on the right in 39.13 is X 2 (1). The last term in 39.13 is

nσ̂2

σ2 =1

σ2

n

∑k=1

(Xk−

(α̂ + β̂ (tk− t̄)

))2

Thus we have

X 2(n)︷ ︸︸ ︷n

∑k=1

(Xk− (α +β (tk− t̄)))2

σ2 =

X 2(1)︷ ︸︸ ︷n

σ2 (α̂−α)2 +

X 2(1)︷ ︸︸ ︷(β̂ −β

)2

σ2 ∑k(tk− t̄)2

+1

σ2

n

∑k=1

(Xk−

(α̂ + β̂ (tk− t̄)

))2(39.15)

In fact, the terms on the right are quadratic forms in the variables Xk− (α +β (tk− t̄))although it does not look like it. Consider the first term.

α̂−α =1n ∑

kXk−α =

1n ∑

k(Xk−α)

=1n ∑

k(Xk− (α +β (ti− t̄)))

This is squared and that is why this term is a quadratic form in the variables

Xk− (α +β (tk− t̄))

Note that the terms added in sum to 0. A similar trick will apply to the other terms. Considerthe second term.

β̂ −β =∑

nk=1 Xk (tk− t̄)

∑k (tk− t̄)2 −β =∑

nk=1 Xk (tk− t̄)−β ∑k (tk− t̄)2

∑k (tk− t̄)2