11.7. HARMONIC FUNCTIONS 279

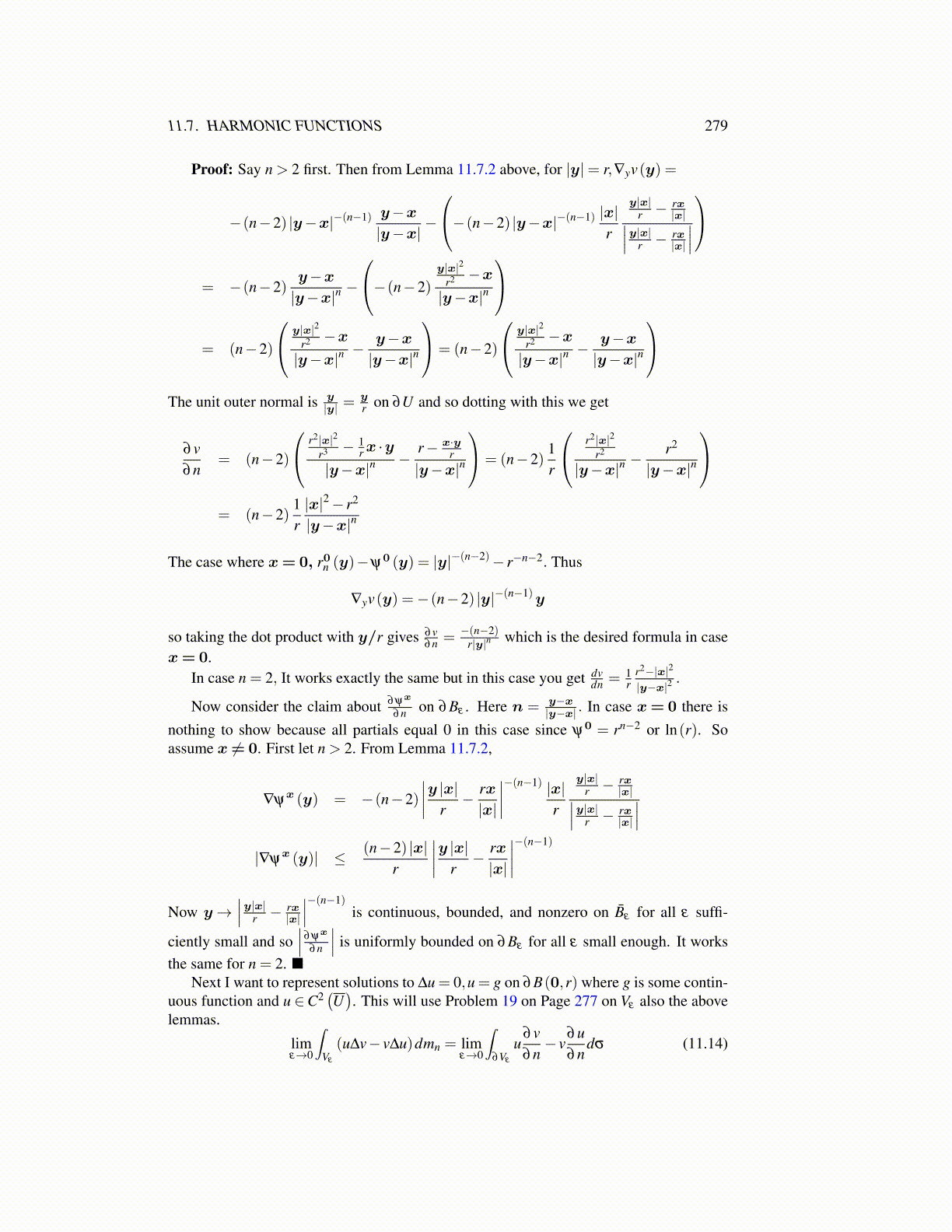
Proof: Say n > 2 first. Then from Lemma 11.7.2 above, for |y|= r,∇yv(y) =

−(n−2) |y−x|−(n−1) y−x

|y−x|−

−(n−2) |y−x|−(n−1) |x|r

y|x|r −

rx|x|∣∣∣y|x|r −rx|x|

∣∣∣

= −(n−2)y−x

|y−x|n−

−(n−2)y|x|2

r2 −x

|y−x|n

= (n−2)

 y|x|2r2 −x

|y−x|n− y−x

|y−x|n

= (n−2)

 y|x|2r2 −x

|y−x|n− y−x

|y−x|n

The unit outer normal is y

|y| =yr on ∂U and so dotting with this we get

∂v∂n

= (n−2)

 r2|x|2r3 − 1

rx ·y|y−x|n

−r− x·y

r|y−x|n

= (n−2)1r

 r2|x|2r2

|y−x|n− r2

|y−x|n

= (n−2)

1r|x|2− r2

|y−x|n

The case where x= 0, r0n (y)−ψ0 (y) = |y|−(n−2)− r−n−2. Thus

∇yv(y) =−(n−2) |y|−(n−1)y

so taking the dot product with y/r gives ∂v∂n = −(n−2)

r|y|n which is the desired formula in casex= 0.

In case n = 2, It works exactly the same but in this case you get dvdn = 1

rr2−|x|2

|y−x|2.

Now consider the claim about ∂ψx

∂n on ∂Bε . Here n = y−x|y−x| . In case x= 0 there is

nothing to show because all partials equal 0 in this case since ψ0 = rn−2 or ln(r). Soassume x ̸= 0. First let n > 2. From Lemma 11.7.2,

∇ψx (y) = −(n−2)

∣∣∣∣y |x|r− rx|x|

∣∣∣∣−(n−1) |x|r

y|x|r −

rx|x|∣∣∣y|x|r −rx|x|

∣∣∣|∇ψ

x (y)| ≤ (n−2) |x|r

∣∣∣∣y |x|r− rx|x|

∣∣∣∣−(n−1)

Now y→∣∣∣y|x|r −

rx|x|

∣∣∣−(n−1)is continuous, bounded, and nonzero on B̄ε for all ε suffi-

ciently small and so∣∣∣ ∂ψx

∂n

∣∣∣ is uniformly bounded on ∂Bε for all ε small enough. It worksthe same for n = 2. ■

Next I want to represent solutions to ∆u = 0,u = g on ∂B(0,r) where g is some contin-uous function and u ∈C2

(U). This will use Problem 19 on Page 277 on Vε also the above

lemmas.

limε→0

∫Vε

(u∆v− v∆u)dmn = limε→0

∫∂Vε

u∂v∂n− v

∂u∂n

dσ (11.14)