268 CHAPTER 11. INTEGRATION ON MANIFOLDS

Indeed, ∂Ω is a closed subset of Ω and so X∂Ω is measurable. That part of the boundarycontained in Ui would then involve a Lebesgue integral over a set of measure zero. Thisshows the following proposition.

Proposition 11.2.4 Let Ω be a differentiable manifold as discussed above and let σ pbe the measure on the manifold defined above. Then σ p (∂Ω) = 0.

Note that it suffices in the above to assume only that DR−1i (u) exists for a.e. u.

11.3 Divergence TheoremThe divergence theorem considered here will feature an open set inRp whose boundary hasa particular form. For convenience, if x ∈ Rp, x̂i ≡

(x1 · · · xi−1 xi+1 · · · xp

)T.

Definition 11.3.1 Let U ⊆ Rp satisfy the following conditions. There exist openboxes, Q1, · · · ,QN , Qi = ∏

pj=1

(ai

j,bij

)such that ∂U ≡U \U is contained in their union.

Also, there exists an open set, Q0 such that Q0 ⊆ Q0 ⊆ U and U ⊆ Q0 ∪Q1 ∪ ·· · ∪QN .Assume for each Qi, there exists k and a function gi such that U ∩Qi is of the form x : (x1, · · · ,xk−1,xk+1, · · · ,xp) ∈∏

k−1j=1

(ai

j,bij

)×

∏pj=k+1

(ai

j,bij

)and ai

k < xk < gi (x1, · · · ,xk−1,xk+1, · · · ,xp)

 (11.8)

or else of the form x : (x1, · · · ,xk−1,xk+1, · · · ,xp) ∈∏k−1j=1

(ai

j,bij

)×

∏pj=k+1

(ai

j,bij

)and gi (x1, · · · ,xk−1,xk+1, · · · ,xp)< xk < bi

j

 (11.9)

The function, gi is differentiable and has a measurable partial derivatives on

Ai ⊆k−1

∏j=1

(ai

j,bij)×

p

∏j=k+1

(ai

j,bij)≡ Q̂k

where

mp−1

(k−1

∏j=1

(ai

j,bij)×

p

∏j=k+1

(ai

j,bij)\Ai

)= 0.

and we assume there is a constant C such that for all i and j,∣∣∣ ∂gi

∂x j

∣∣∣≤C off Ai and that eachgi is Lipschitz. Thus there are no measurability issues by Theorem 10.3.1.

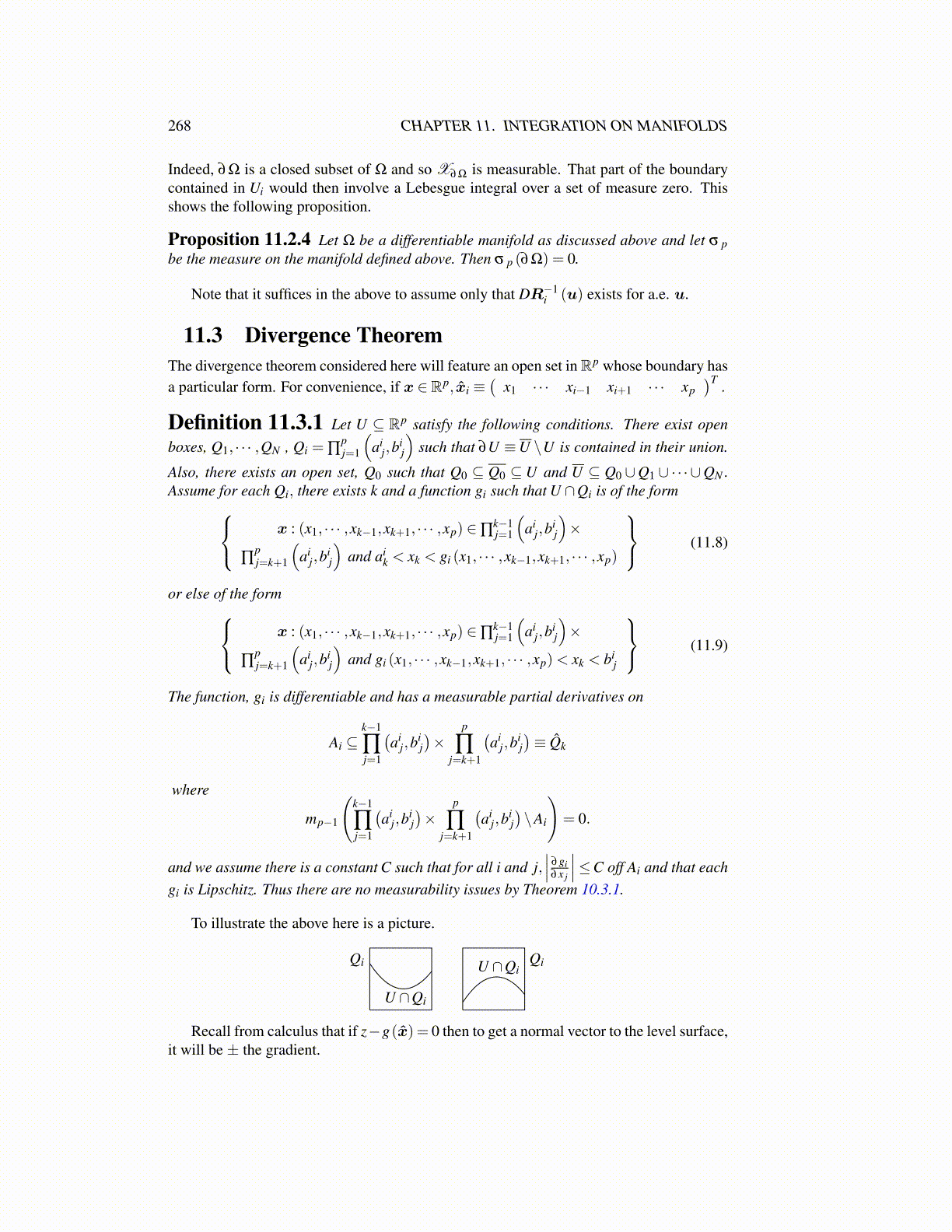
To illustrate the above here is a picture.

U ∩Qi

Qi U ∩QiQi

Recall from calculus that if z−g(x̂) = 0 then to get a normal vector to the level surface,it will be ± the gradient.