C.1. THE SPINNING TOP AND THE EULER ANGLES 883
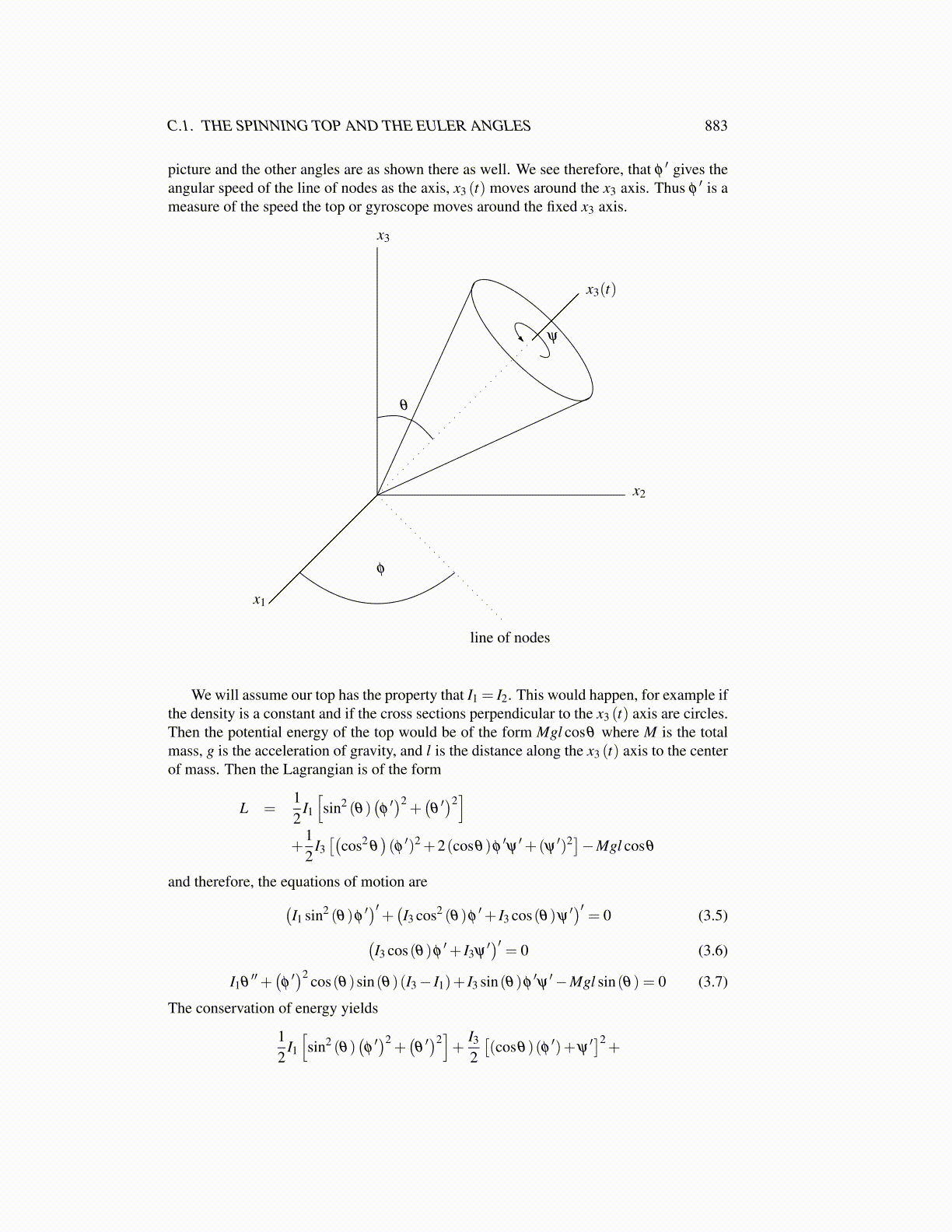
picture and the other angles are as shown there as well. We see therefore, that φ′ gives the
angular speed of the line of nodes as the axis, x3 (t) moves around the x3 axis. Thus φ′ is a
measure of the speed the top or gyroscope moves around the fixed x3 axis.
x1
x2
x3
ψ
x3(t)
φ
line of nodes
θ
We will assume our top has the property that I1 = I2. This would happen, for example ifthe density is a constant and if the cross sections perpendicular to the x3 (t) axis are circles.Then the potential energy of the top would be of the form Mgl cosθ where M is the totalmass, g is the acceleration of gravity, and l is the distance along the x3 (t) axis to the centerof mass. Then the Lagrangian is of the form
L =12
I1
[sin2 (θ)
(φ′)2
+(θ′)2]
+12
I3[(
cos2θ)(φ ′)2 +2(cosθ)φ
′ψ′+(ψ ′)2]−Mgl cosθ
and therefore, the equations of motion are(I1 sin2 (θ)φ
′)′+ (I3 cos2 (θ)φ′+ I3 cos(θ)ψ
′)′ = 0 (3.5)(I3 cos(θ)φ
′+ I3ψ′)′ = 0 (3.6)
I1θ′′+(φ′)2 cos(θ)sin(θ)(I3− I1)+ I3 sin(θ)φ
′ψ′−Mgl sin(θ) = 0 (3.7)
The conservation of energy yields
12
I1
[sin2 (θ)
(φ′)2
+(θ′)2]+
I3
2[(cosθ)(φ ′)+ψ
′]2+