832 CHAPTER 39. STATISTICAL TESTS

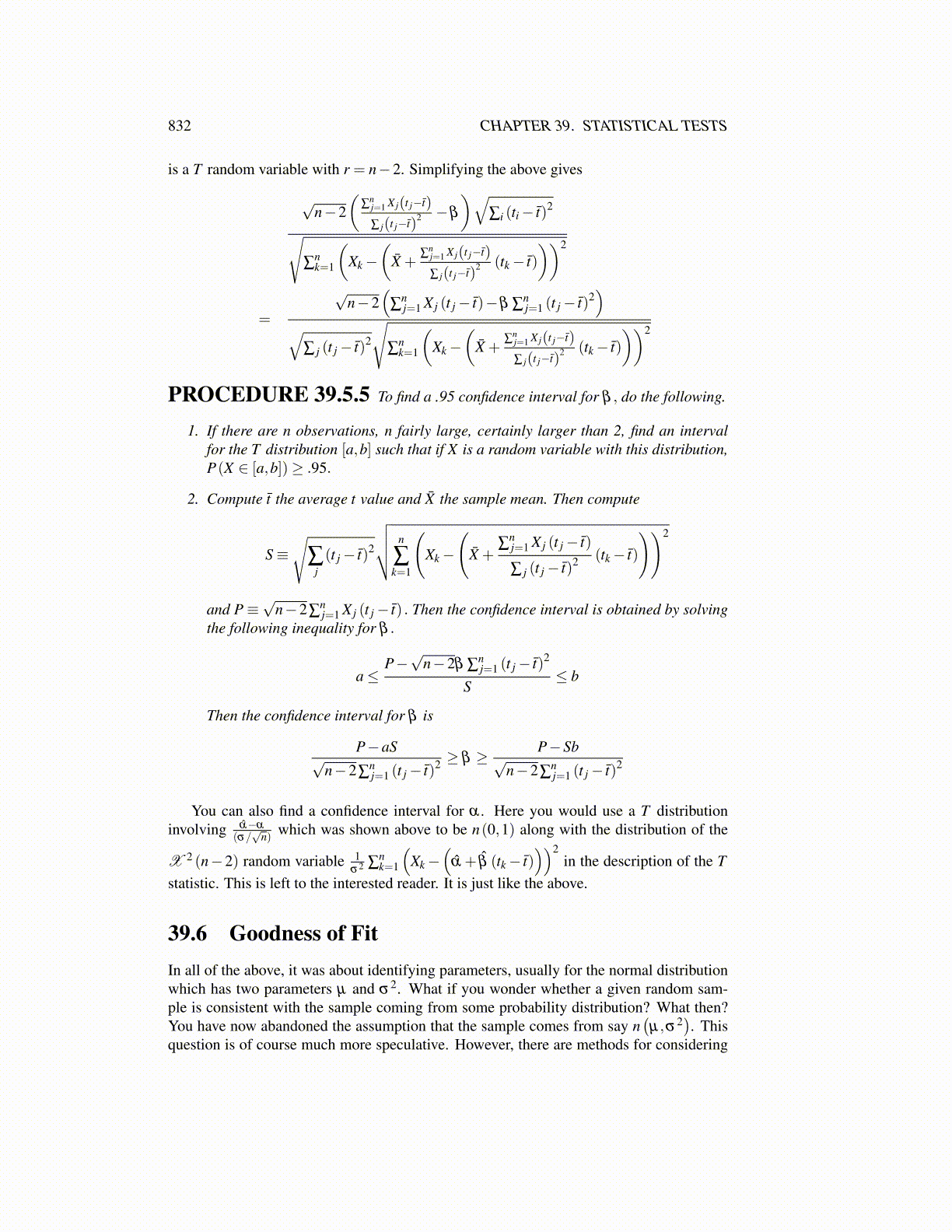
is a T random variable with r = n−2. Simplifying the above gives

√n−2

(∑

nj=1 X j(t j−t̄)

∑ j(t j−t̄)2 −β

)√∑i (ti− t̄)2√

∑nk=1

(Xk−

(X̄ +

∑nj=1 X j(t j−t̄)

∑ j(t j−t̄)2 (tk− t̄)

))2

=

√n−2

(∑

nj=1 X j (t j− t̄)−β ∑

nj=1 (t j− t̄)2

)√

∑ j (t j− t̄)2

√∑

nk=1

(Xk−

(X̄ +

∑nj=1 X j(t j−t̄)

∑ j(t j−t̄)2 (tk− t̄)

))2

PROCEDURE 39.5.5 To find a .95 confidence interval for β , do the following.

1. If there are n observations, n fairly large, certainly larger than 2, find an intervalfor the T distribution [a,b] such that if X is a random variable with this distribution,P(X ∈ [a,b])≥ .95.

2. Compute t̄ the average t value and X̄ the sample mean. Then compute

S≡√

∑j(t j− t̄)2

√√√√ n

∑k=1

(Xk−

(X̄ +

∑nj=1 X j (t j− t̄)

∑ j (t j− t̄)2 (tk− t̄)

))2

and P≡√

n−2∑nj=1 X j (t j− t̄) . Then the confidence interval is obtained by solving

the following inequality for β .

a≤P−√

n−2β ∑nj=1 (t j− t̄)2

S≤ b

Then the confidence interval for β is

P−aS√

n−2∑nj=1 (t j− t̄)2 ≥ β ≥ P−Sb

√n−2∑

nj=1 (t j− t̄)2

You can also find a confidence interval for α . Here you would use a T distributioninvolving α̂−α

(σ/√

n) which was shown above to be n(0,1) along with the distribution of the

X 2 (n−2) random variable 1σ2 ∑

nk=1

(Xk−

(α̂ + β̂ (tk− t̄)

))2in the description of the T

statistic. This is left to the interested reader. It is just like the above.

39.6 Goodness of FitIn all of the above, it was about identifying parameters, usually for the normal distributionwhich has two parameters µ and σ2. What if you wonder whether a given random sam-ple is consistent with the sample coming from some probability distribution? What then?You have now abandoned the assumption that the sample comes from say n

(µ,σ2

). This

question is of course much more speculative. However, there are methods for considering