3.2. UNIFORM CONVERGENCE OF CONTINUOUS FUNCTIONS 43
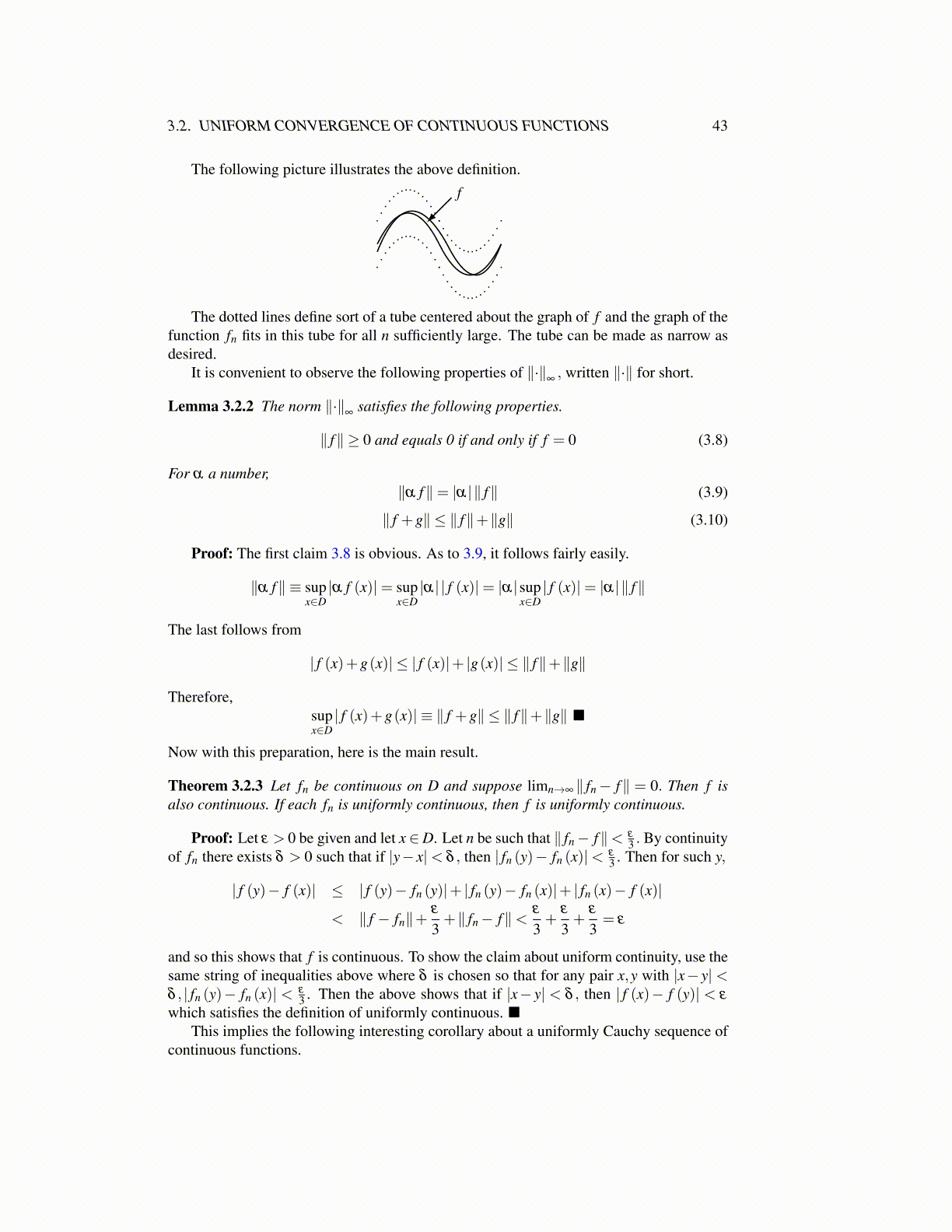
The following picture illustrates the above definition.
f
The dotted lines define sort of a tube centered about the graph of f and the graph of thefunction fn fits in this tube for all n sufficiently large. The tube can be made as narrow asdesired.
It is convenient to observe the following properties of ∥·∥∞, written ∥·∥ for short.
Lemma 3.2.2 The norm ∥·∥∞
satisfies the following properties.
∥ f∥ ≥ 0 and equals 0 if and only if f = 0 (3.8)
For α a number,∥α f∥= |α|∥ f∥ (3.9)
∥ f +g∥ ≤ ∥ f∥+∥g∥ (3.10)
Proof: The first claim 3.8 is obvious. As to 3.9, it follows fairly easily.
∥α f∥ ≡ supx∈D|α f (x)|= sup
x∈D|α| | f (x)|= |α|sup
x∈D| f (x)|= |α|∥ f∥
The last follows from
| f (x)+g(x)| ≤ | f (x)|+ |g(x)| ≤ ∥ f∥+∥g∥
Therefore,supx∈D| f (x)+g(x)| ≡ ∥ f +g∥ ≤ ∥ f∥+∥g∥ ■
Now with this preparation, here is the main result.
Theorem 3.2.3 Let fn be continuous on D and suppose limn→∞ ∥ fn− f∥ = 0. Then f isalso continuous. If each fn is uniformly continuous, then f is uniformly continuous.
Proof: Let ε > 0 be given and let x∈D. Let n be such that ∥ fn− f∥< ε
3 . By continuityof fn there exists δ > 0 such that if |y− x|< δ , then | fn (y)− fn (x)|< ε
3 . Then for such y,
| f (y)− f (x)| ≤ | f (y)− fn (y)|+ | fn (y)− fn (x)|+ | fn (x)− f (x)|
< ∥ f − fn∥+ε
3+∥ fn− f∥< ε
3+
ε
3+
ε
3= ε
and so this shows that f is continuous. To show the claim about uniform continuity, use thesame string of inequalities above where δ is chosen so that for any pair x,y with |x− y| <δ , | fn (y)− fn (x)| < ε
3 . Then the above shows that if |x− y| < δ , then | f (x)− f (y)| < ε
which satisfies the definition of uniformly continuous. ■This implies the following interesting corollary about a uniformly Cauchy sequence of
continuous functions.