3.1. PROPERTIES OF THE INTEGRAL 41

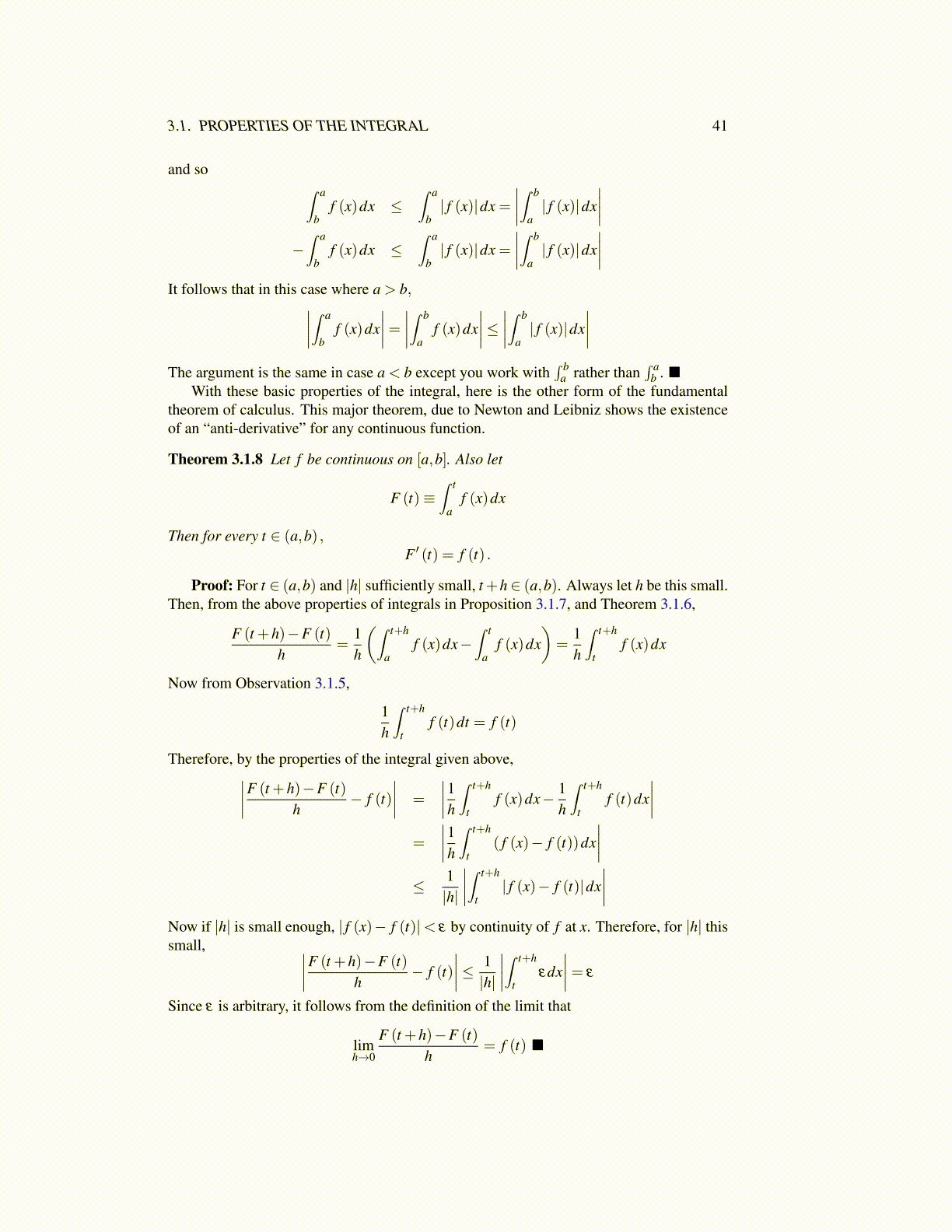
and so ∫ a

bf (x)dx ≤

∫ a

b| f (x)|dx =

∣∣∣∣∫ b

a| f (x)|dx

∣∣∣∣−∫ a

bf (x)dx ≤

∫ a

b| f (x)|dx =

∣∣∣∣∫ b

a| f (x)|dx

∣∣∣∣It follows that in this case where a > b,∣∣∣∣∫ a

bf (x)dx

∣∣∣∣= ∣∣∣∣∫ b

af (x)dx

∣∣∣∣≤ ∣∣∣∣∫ b

a| f (x)|dx

∣∣∣∣The argument is the same in case a < b except you work with

∫ ba rather than

∫ ab . ■

With these basic properties of the integral, here is the other form of the fundamentaltheorem of calculus. This major theorem, due to Newton and Leibniz shows the existenceof an “anti-derivative” for any continuous function.

Theorem 3.1.8 Let f be continuous on [a,b]. Also let

F (t)≡∫ t

af (x)dx

Then for every t ∈ (a,b) ,F ′ (t) = f (t) .

Proof: For t ∈ (a,b) and |h| sufficiently small, t+h∈ (a,b). Always let h be this small.Then, from the above properties of integrals in Proposition 3.1.7, and Theorem 3.1.6,

F (t +h)−F (t)h

=1h

(∫ t+h

af (x)dx−

∫ t

af (x)dx

)=

1h

∫ t+h

tf (x)dx

Now from Observation 3.1.5,

1h

∫ t+h

tf (t)dt = f (t)

Therefore, by the properties of the integral given above,∣∣∣∣F (t +h)−F (t)h

− f (t)∣∣∣∣ =

∣∣∣∣1h∫ t+h

tf (x)dx− 1

h

∫ t+h

tf (t)dx

∣∣∣∣=

∣∣∣∣1h∫ t+h

t( f (x)− f (t))dx

∣∣∣∣≤ 1|h|

∣∣∣∣∫ t+h

t| f (x)− f (t)|dx

∣∣∣∣Now if |h| is small enough, | f (x)− f (t)|< ε by continuity of f at x. Therefore, for |h| thissmall, ∣∣∣∣F (t +h)−F (t)

h− f (t)

∣∣∣∣≤ 1|h|

∣∣∣∣∫ t+h

tεdx∣∣∣∣= ε

Since ε is arbitrary, it follows from the definition of the limit that

limh→0

F (t +h)−F (t)h

= f (t) ■