12 CHAPTER 1. SOME PREREQUISITE TOPICS
-50 0 50
x
-50
0
50
y
-2 0 2
x
-2
0
2
y
-4 -2 0 2 4 6
x
-2
0
2
4
y
r too big r too small r just right
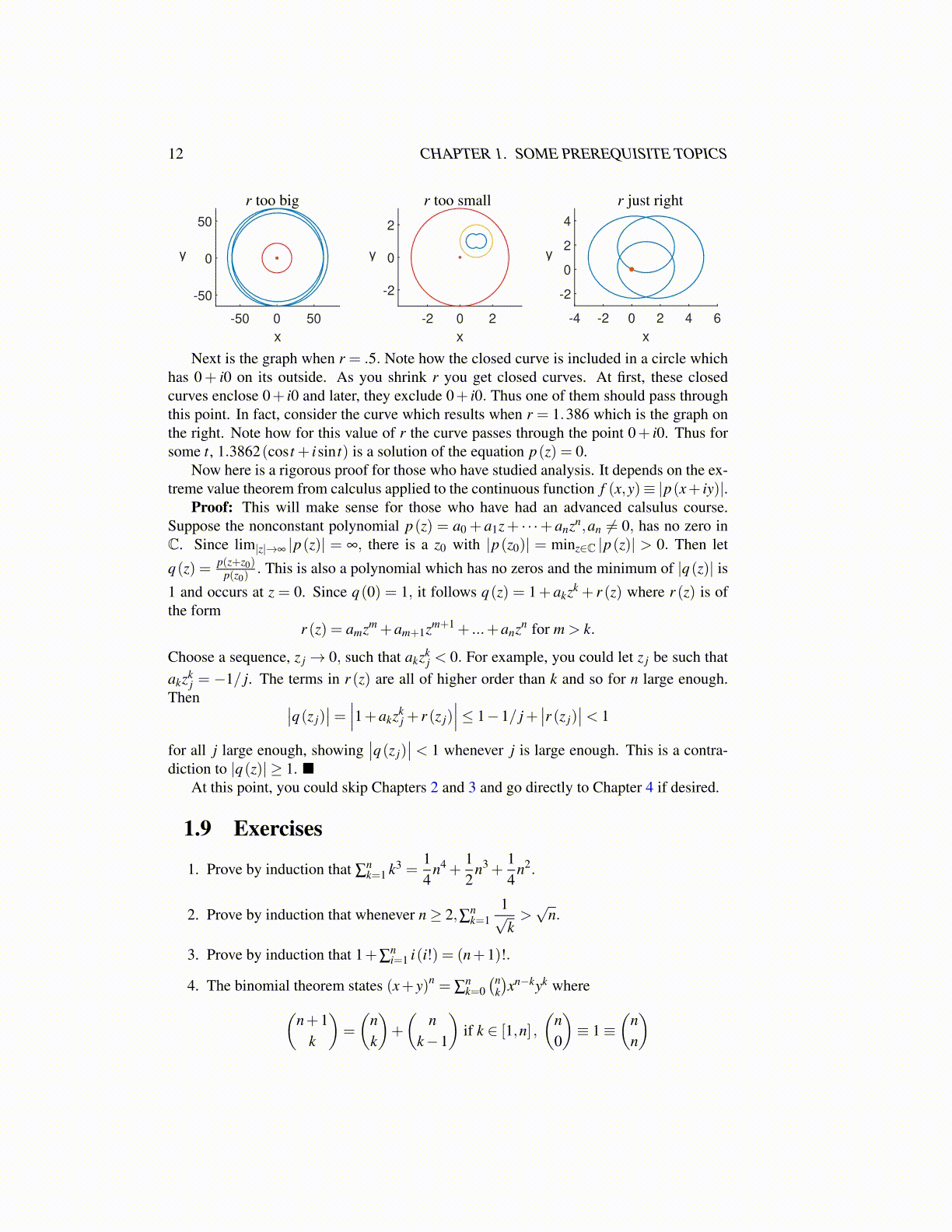
Next is the graph when r = .5. Note how the closed curve is included in a circle whichhas 0+ i0 on its outside. As you shrink r you get closed curves. At first, these closedcurves enclose 0+ i0 and later, they exclude 0+ i0. Thus one of them should pass throughthis point. In fact, consider the curve which results when r = 1.386 which is the graph onthe right. Note how for this value of r the curve passes through the point 0+ i0. Thus forsome t, 1.3862(cos t + isin t) is a solution of the equation p(z) = 0.
Now here is a rigorous proof for those who have studied analysis. It depends on the ex-treme value theorem from calculus applied to the continuous function f (x,y)≡ |p(x+ iy)|.
Proof: This will make sense for those who have had an advanced calsulus course.Suppose the nonconstant polynomial p(z) = a0 + a1z+ · · ·+ anzn,an ̸= 0, has no zero inC. Since lim|z|→∞ |p(z)| = ∞, there is a z0 with |p(z0)| = minz∈C |p(z)| > 0. Then let
q(z) = p(z+z0)p(z0)
. This is also a polynomial which has no zeros and the minimum of |q(z)| is1 and occurs at z = 0. Since q(0) = 1, it follows q(z) = 1+ akzk + r (z) where r (z) is ofthe form
r (z) = amzm +am+1zm+1 + ...+anzn for m > k.
Choose a sequence, z j → 0, such that akzkj < 0. For example, you could let z j be such that
akzkj = −1/ j. The terms in r (z) are all of higher order than k and so for n large enough.
Then ∣∣q(z j)∣∣= ∣∣∣1+akzk
j + r (z j)∣∣∣≤ 1−1/ j+
∣∣r (z j)∣∣< 1
for all j large enough, showing∣∣q(z j)
∣∣ < 1 whenever j is large enough. This is a contra-diction to |q(z)| ≥ 1. ■
At this point, you could skip Chapters 2 and 3 and go directly to Chapter 4 if desired.
1.9 Exercises
1. Prove by induction that ∑nk=1 k3 =
14
n4 +12
n3 +14
n2.
2. Prove by induction that whenever n≥ 2,∑nk=1
1√k>√
n.
3. Prove by induction that 1+∑ni=1 i(i!) = (n+1)!.
4. The binomial theorem states (x+ y)n = ∑nk=0(n
k
)xn−kyk where(
n+1k
)=
(nk
)+
(n
k−1
)if k ∈ [1,n] ,
(n0
)≡ 1≡
(nn
)