438 CHAPTER 17. SPECTRAL THEORY OF LINEAR MAPS

To get the higher derivatives, note S (t) has infinitely many derivatives due to t being acomplex variable. Therefore,

S′′ (t) = limh→0

S′ (t +h)−S′ (t)h

= limh→0

AS (t +h)−S (t)

h

and S(t+h)−S(t)h → AS (t) and so since A is closed, AS (t) ∈ D(A) and the above becomes

A2S (t). Continuing this way yields the claims 1.) and 2.). Note this also implies S (t)x ∈D(A) for each t ∈ Sr which says more than S (t)x ∈ H. In practice this has the effect ofregularizing the solution to an initial value problem.

Next consider the semigroup property. Let s, t ∈ Sr. As described above let γ ′ denotethe contour shifted slightly to the right. Then

S (t)S (s) =(

12πi

)2 ∫γ

∫γ ′

eλ t (λ I−A)−1 eµs (µI−A)−1 dµdλ (17.29)

Using the resolvent identity,

(λ I−A)−1 (µI−A)−1 = (µ−λ )−1((λ I−A)−1− (µI−A)−1

),

then substituting this resolvent identity in 17.29, it equals(1

2πi

)2 ∫γ

∫γ ′

eµseλ t((µ−λ )−1

((λ I−A)−1− (µI−A)−1

))dµdλ

= −(

12πi

)2 ∫γ

eλ t∫

γ ′eµs (µ−λ )−1 (µI−A)−1 dµdλ

+

(1

2πi

)2 ∫γ

∫γ ′

eµseλ t (µ−λ )−1 (λ I−A)−1 dµdλ

The order of integration can be interchanged because of the absolute convergence and Fu-bini’s theorem. Then this reduces to

= −(

12πi

)2 ∫γ ′(µI−A)−1 eµs

∫γ

eλ t (µ−λ )−1 dλdµ

+

(1

2πi

)2 ∫γ

(λ I−A)−1 eλ t∫

γ ′eµs (µ−λ )−1 dµdλ

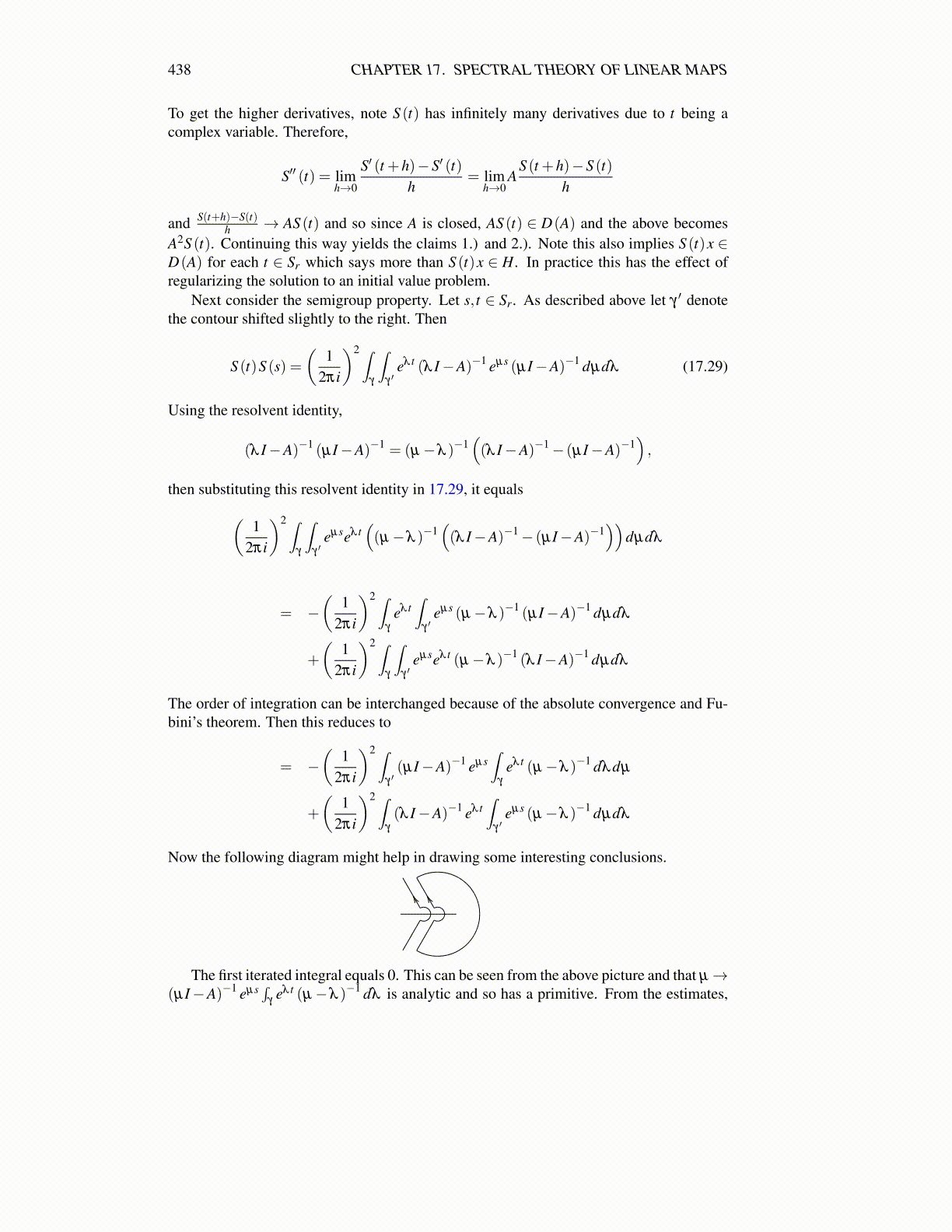
Now the following diagram might help in drawing some interesting conclusions.

The first iterated integral equals 0. This can be seen from the above picture and that µ→(µI−A)−1 eµs ∫

γeλ t (µ−λ )−1 dλ is analytic and so has a primitive. From the estimates,