17.4. SECTORIAL OPERATORS AND ANALYTIC SEMIGROUPS 433

The radius of the little circle is not important because the functions to be integrated willbe analytic on Saφ .

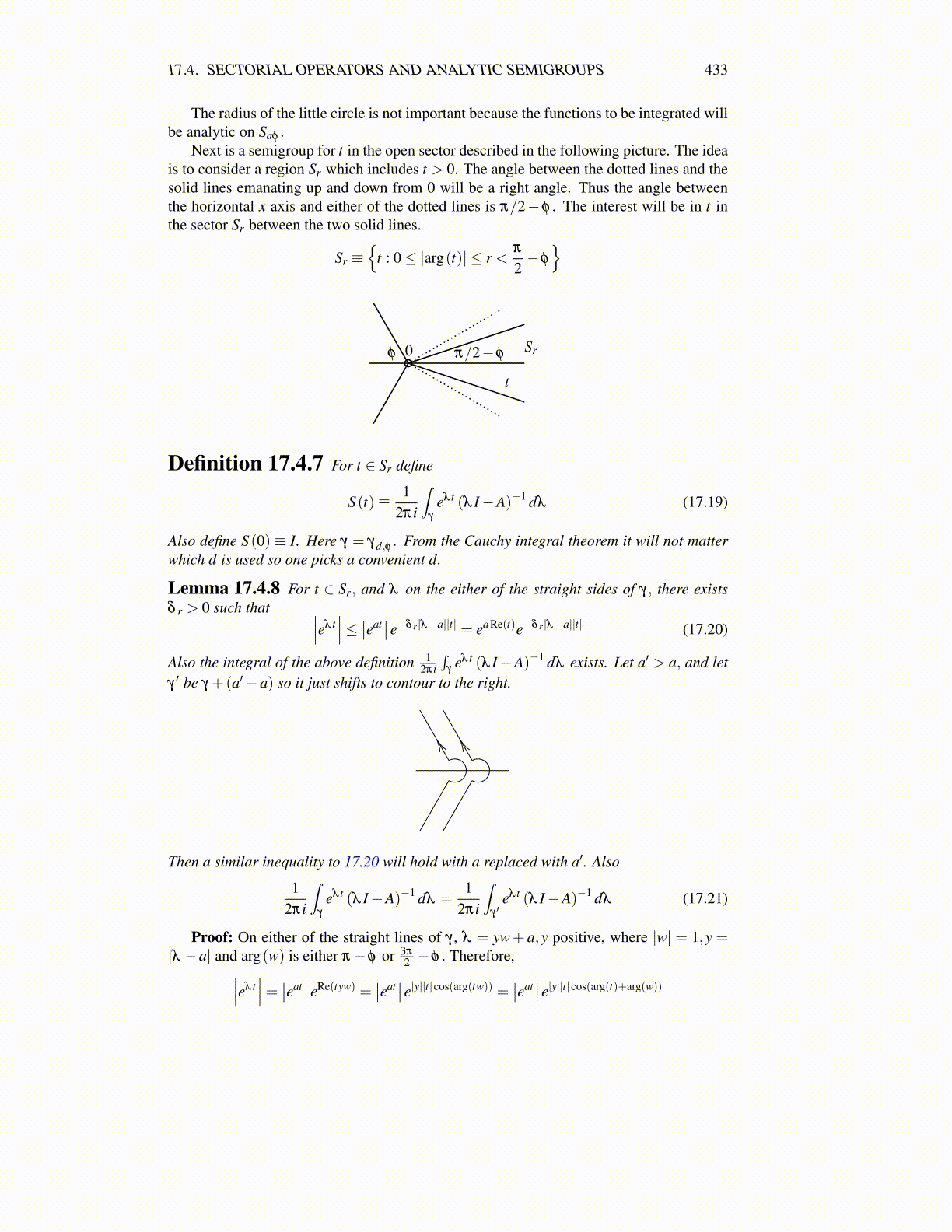
Next is a semigroup for t in the open sector described in the following picture. The ideais to consider a region Sr which includes t > 0. The angle between the dotted lines and thesolid lines emanating up and down from 0 will be a right angle. Thus the angle betweenthe horizontal x axis and either of the dotted lines is π/2−φ . The interest will be in t inthe sector Sr between the two solid lines.

Sr ≡{

t : 0≤ |arg(t)| ≤ r <π

2−φ

}

0

t

φ π/2−φSr

Definition 17.4.7 For t ∈ Sr define

S (t)≡ 12πi

∫γ

eλ t (λ I−A)−1 dλ (17.19)

Also define S (0) ≡ I. Here γ = γd,φ . From the Cauchy integral theorem it will not matterwhich d is used so one picks a convenient d.

Lemma 17.4.8 For t ∈ Sr, and λ on the either of the straight sides of γ, there existsδ r > 0 such that ∣∣∣eλ t

∣∣∣≤ ∣∣eat ∣∣e−δ r |λ−a||t| = eaRe(t)e−δ r |λ−a||t| (17.20)

Also the integral of the above definition 12πi∫

γeλ t (λ I−A)−1 dλ exists. Let a′ > a, and let

γ ′ be γ +(a′−a) so it just shifts to contour to the right.

Then a similar inequality to 17.20 will hold with a replaced with a′. Also

12πi

∫γ

eλ t (λ I−A)−1 dλ =1

2πi

∫γ ′

eλ t (λ I−A)−1 dλ (17.21)

Proof: On either of the straight lines of γ , λ = yw+ a,y positive, where |w| = 1,y =|λ −a| and arg(w) is either π−φ or 3π

2 −φ . Therefore,∣∣∣eλ t∣∣∣= ∣∣eat ∣∣eRe(tyw) =

∣∣eat ∣∣e|y||t|cos(arg(tw)) =∣∣eat ∣∣e|y||t|cos(arg(t)+arg(w))