17.2. FUNCTIONS OF LINEAR TRANSFORMATIONS 425
Now using the Fubini theorem, Theorem 14.4.9,
=∫
Γ
f (λ )(λ I−A)−1∫
Γ̂
g(µ)1
µ−λdµdλ +
∫Γ̂
g(µ)(µI−A)−1∫
Γ
f (λ )1
λ −µdλdµ
The first term is 0 from Cauchy’s integral formula, Theorem 14.11.1 applied to the indi-vidual simple closed curves whose union is Γ because the winding number n
(Γ̂,λ
)= 0.
Thus the inside integral vanishes. From the Cauchy integral formula, the second term is2πi
∫Γ̂
g(µ)(µI−A)−1 f (µ)dµ and so
f (A)g(A) =1
2πi
∫Γ̂
f (µ)g(µ)(µI−A)−1 dµ =1
2πi
∫Γ
f (λ )g(λ )(λ I−A)−1 dλ ■
Now consider the case that f (λ ) = λ . Is f (A), defined in terms of integrals as above,equal to A? If so, then from the theorem just shown, λ
n used in the integral formula doeslead to An. Thus one considers 1
2πi∫
Γλ (λ I−A)−1 dλ where Γ is a cycle such that n(Γ,z)=
1 if z ∈ σ (A), n(Γ,z) is an integer if z ∈Ω, and n(Γ,z) = 0 if z /∈Ω,Γ∗∩σ (A) = /0.Let Ω̂≡ σ (A)C and let γ̂R be a large circle of radius R > ∥A∥ oriented clockwise, which
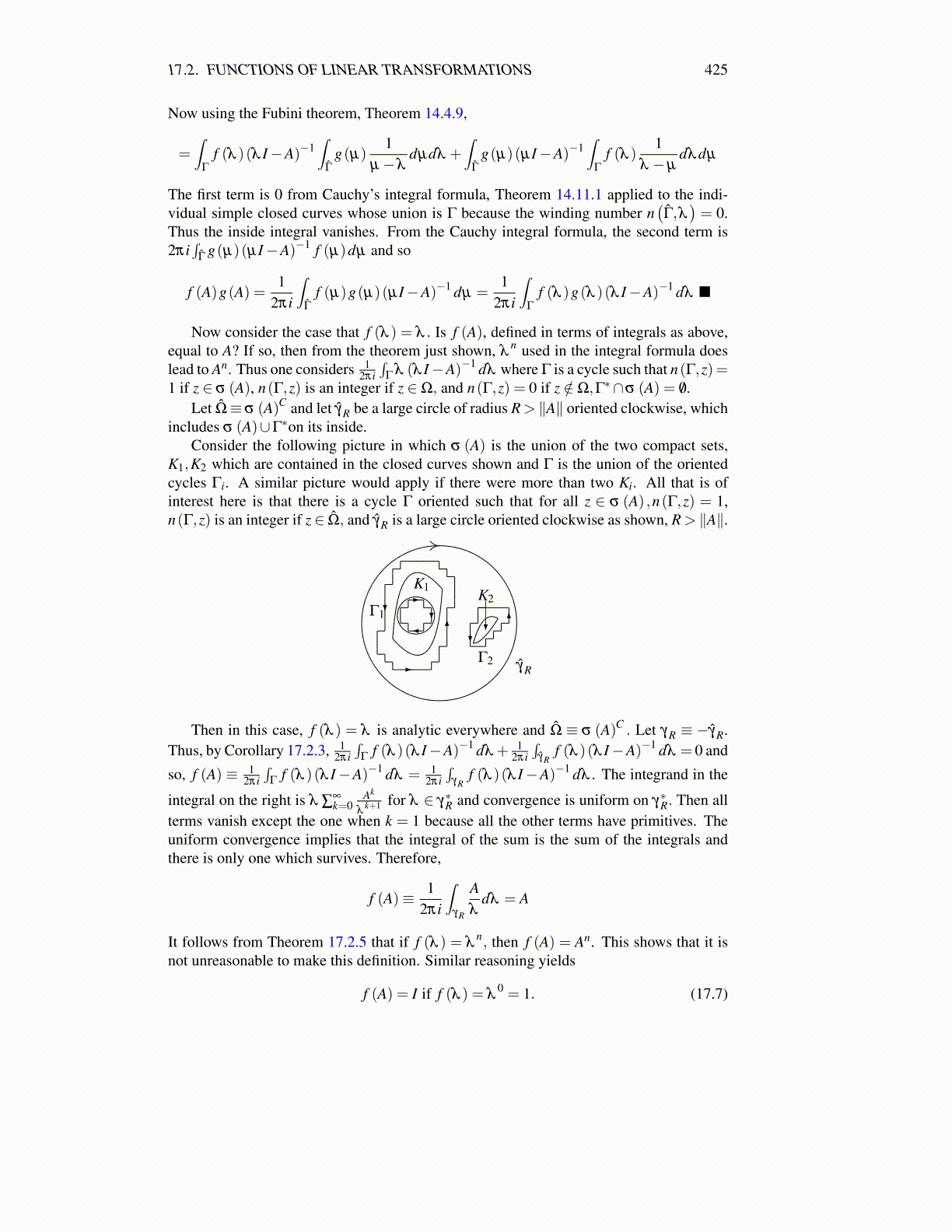
includes σ (A)∪Γ∗on its inside.Consider the following picture in which σ (A) is the union of the two compact sets,
K1,K2 which are contained in the closed curves shown and Γ is the union of the orientedcycles Γi. A similar picture would apply if there were more than two Ki. All that is ofinterest here is that there is a cycle Γ oriented such that for all z ∈ σ (A) ,n(Γ,z) = 1,n(Γ,z) is an integer if z∈ Ω̂, and γ̂R is a large circle oriented clockwise as shown, R > ∥A∥.
γ̂R
K1
Γ1
K2
Γ2
Then in this case, f (λ ) = λ is analytic everywhere and Ω̂ ≡ σ (A)C . Let γR ≡ −γ̂R.Thus, by Corollary 17.2.3, 1
2πi∫
Γf (λ )(λ I−A)−1 dλ + 1
2πi∫
γ̂Rf (λ )(λ I−A)−1 dλ = 0 and
so, f (A) ≡ 12πi∫
Γf (λ )(λ I−A)−1 dλ = 1
2πi∫
γRf (λ )(λ I−A)−1 dλ . The integrand in the
integral on the right is λ ∑∞k=0
Ak
λk+1 for λ ∈ γ∗R and convergence is uniform on γ∗R. Then all
terms vanish except the one when k = 1 because all the other terms have primitives. Theuniform convergence implies that the integral of the sum is the sum of the integrals andthere is only one which survives. Therefore,
f (A)≡ 12πi
∫γR
Aλ
dλ = A
It follows from Theorem 17.2.5 that if f (λ ) = λn, then f (A) = An. This shows that it is
not unreasonable to make this definition. Similar reasoning yields
f (A) = I if f (λ ) = λ0 = 1. (17.7)