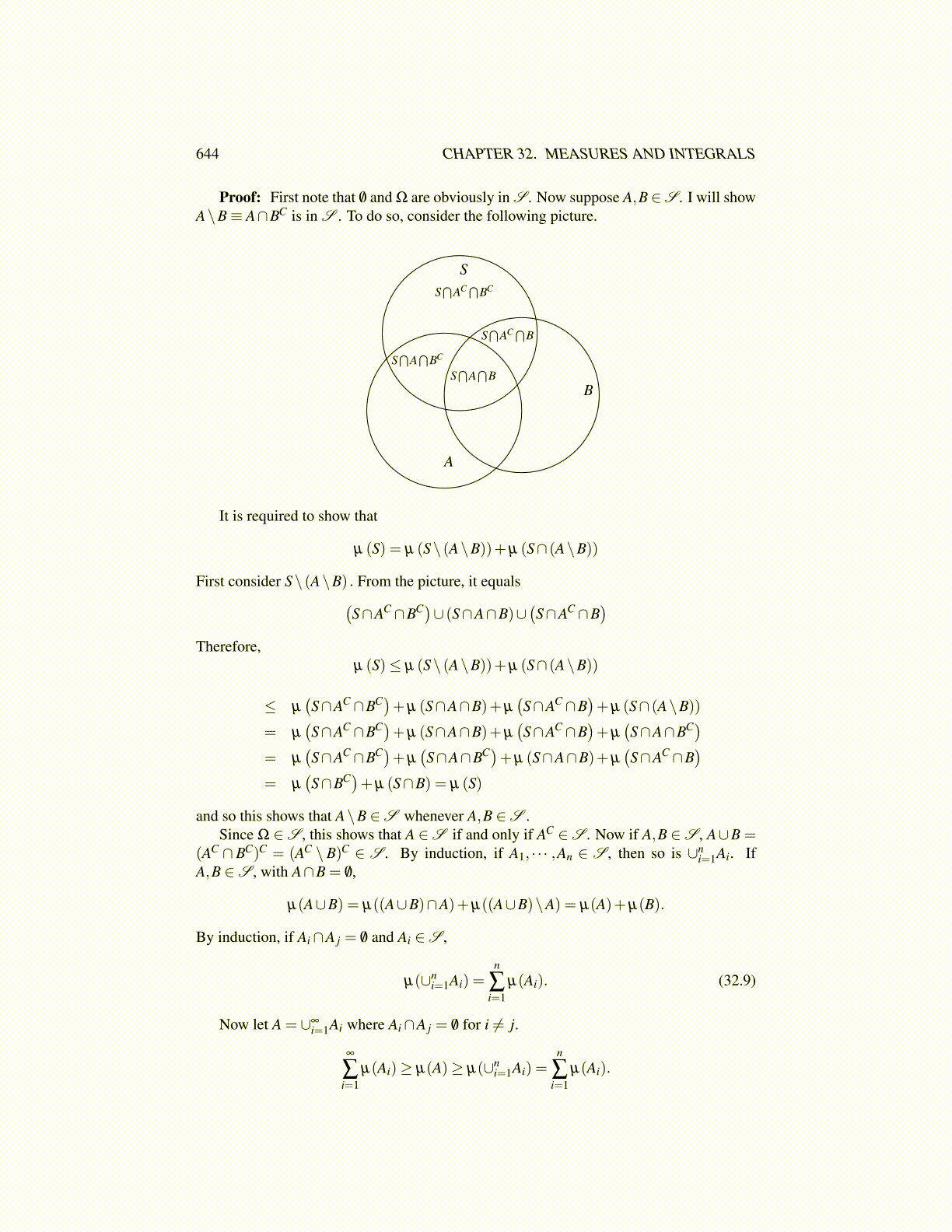
644 CHAPTER 32. MEASURES AND INTEGRALS
Definition 32.6.1 Let (Ω,F , µ) be a measure space and suppose f : Ω → [0,∞] ismeasurable. Then define∫
f dµ ≡∫
∞
0µ ([ f > λ ])dλ =
∫∞
0µ(
f−1 (λ ,∞))
dλ
which makes sense because λ → µ ([ f > λ ]) is nonnegative and decreasing. On the rightyou have an improper Riemann integral like what was discussed above.
Note that if f ≤ g, then∫
f dµ ≤∫
gdµ because µ ([ f > λ ])≤ µ ([g > λ ]) . Next I pointout that the integral is a limit of lower sums.
Lemma 32.6.2 In the situation of the above definition,∫f dµ = sup
h>0
∞
∑i=1
µ ([ f > hi])h
Proof: Let m(h,R) ∈ N satisfy R−h < hm(h,R)≤ R. Then
limR→∞
m(h,R) = ∞
and so ∫f dµ ≡
∫∞
0µ ([ f > λ ])dλ = sup
Msup
R
∫ R
0µ ([ f > λ ])∧Mdλ =
supM
supR>0
suph>0
m(h,R)
∑k=1
(µ ([ f > kh])∧M)h+(µ ([ f > R])∧M)(R−hm(h,R)) (32.11)
= supM
supR>0
suph>0
m(h,R)
∑k=1
(µ ([ f > kh])∧M)h
because the sum in 32.11 is just a lower sum for the integral∫ R
0 µ ([ f > λ ])∧Mdλ , theselower sums are increasing, and the last term is smaller than Mh. Hence, switching the orderof the sups, this equals
supR>0
suph>0
supM
m(h,R)
∑k=1
(µ ([ f > kh])∧M)h = supR>0
suph>0
limM→∞
m(h,R)
∑k=1
(µ ([ f > kh])∧M)h
= suph>0
supR
m(R,h)
∑k=1
(µ ([ f > kh]))h = suph>0
∞
∑k=1
(µ ([ f > kh]))h.
32.7 Nonnegative Simple FunctionsTo begin with, here is a useful lemma.
Lemma 32.7.1 If f (λ ) = 0 for all λ > a, where f is a decreasing nonnegative function,then ∫
∞
0f (λ )dλ =
∫ a
0f (λ )dλ .