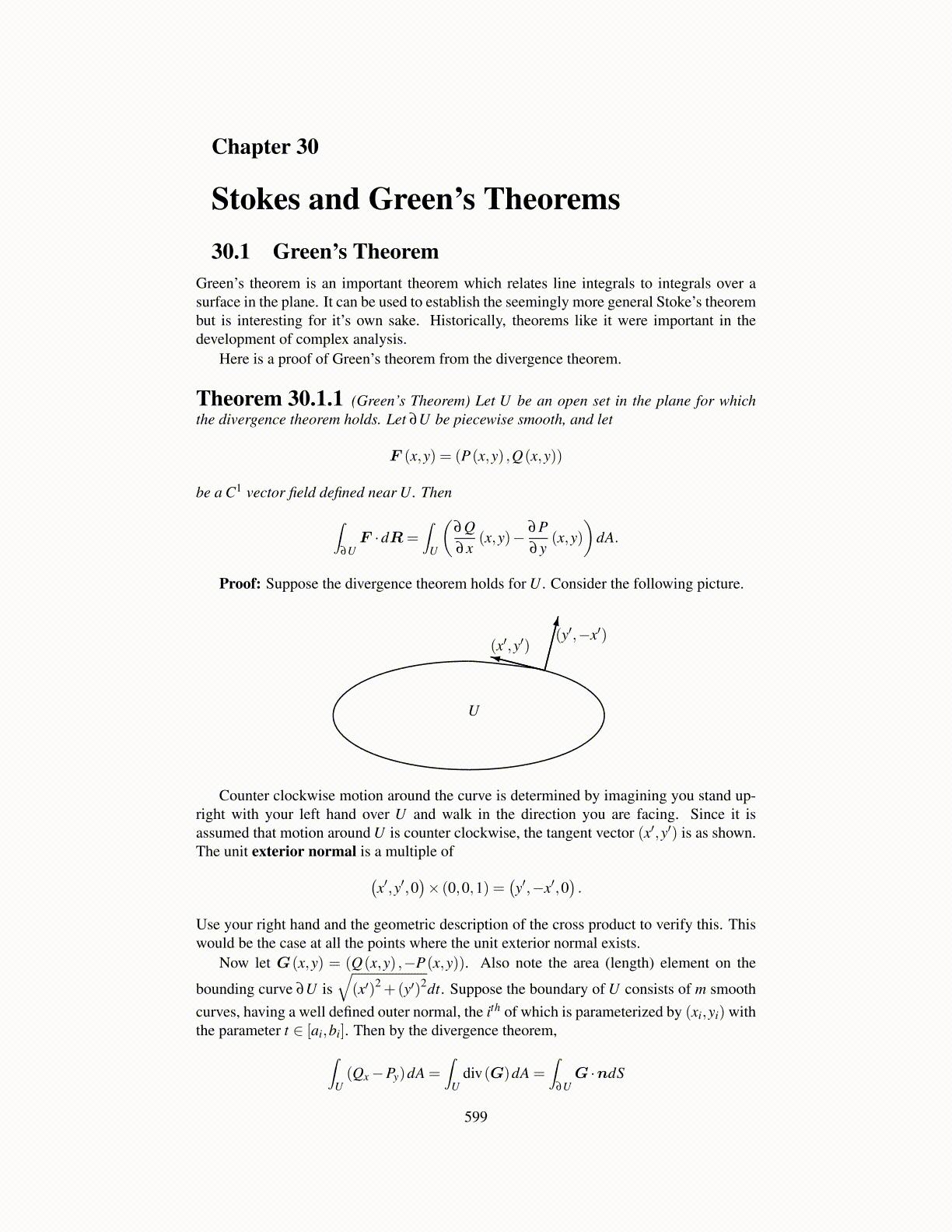
30.3. STOKE’S THEOREM FROM GREEN’S THEOREM 599

Recall the following definition of the curl of a vector field. Why do we even considerit?

Definition 30.3.1 Let F (x,y,z) = (F1 (x,y,z) ,F2 (x,y,z) ,F3 (x,y,z)) be a C1 vectorfield defined on an open set V in R3. Then

∇×F ≡

∣∣∣∣∣∣i j k∂

∂x∂

∂y∂

∂ zF1 F2 F3

∣∣∣∣∣∣≡(

∂F3

∂y− ∂F2

∂ z

)i+

(∂F1

∂ z− ∂F3

∂x

)j+

(∂F2

∂x− ∂F1

∂y

)k.

This is also called curl(F ) and written as indicated, ∇×F .

The following lemma gives the fundamental identity which will be used in the proof ofStoke’s theorem.

Lemma 30.3.2 Let R : U →V ⊆R3 where U is an open subset of R2 and V is an opensubset of R3. Suppose R is C2 and let F be a C1 vector field defined in V .

(Ru ×Rv) · (∇×F )(R(u,v)) = ((F ◦R)u ·Rv − (F ◦R)v ·Ru)(u,v) . (30.1)

Proof: Start with the left side and let xi = Ri (u,v) for short.

(Ru ×Rv) · (∇×F )(R(u,v)) = ε i jkx juxkvε irs∂Fs

∂xr= (δ jrδ ks −δ jsδ kr)x juxkv

∂Fs

∂xr

= x juxkv∂Fk

∂x j− x juxkv

∂Fj

∂xk=Rv ·

∂ (F ◦R)

∂u−Ru ·

∂ (F ◦R)

∂v

which proves 30.1.The proof of Stoke’s theorem given next follows [10]. First, it is convenient to give a

definition.

Definition 30.3.3 A vector valued function R : U ⊆ Rm → Rn is said to be inCk(U ,Rn

)if it is the restriction to U of a vector valued function which is defined on Rm

and is Ck. That is, this function has continuous partial derivatives up to order k.

Theorem 30.3.4 (Stoke’s Theorem) Let U be any region in R2 for which the con-clusion of Green’s theorem holds and let R∈C2

(U ,R3

)be a one to one function satisfying

|(Ru ×Rv)(u,v)| ̸= 0 for all (u,v) ∈U and let S denote the surface

S ≡ {R(u,v) : (u,v) ∈U} , ∂S ≡ {R(u,v) : (u,v) ∈ ∂U}

where the orientation on ∂S is consistent with the counter clockwise orientation on ∂U (Uis on the left as you walk around ∂U). Then for F a C1 vector field defined near S,∫

∂SF ·dR=

∫S

curl(F ) ·ndS

where n is the normal to S defined by

n≡ Ru ×Rv

|Ru ×Rv|.