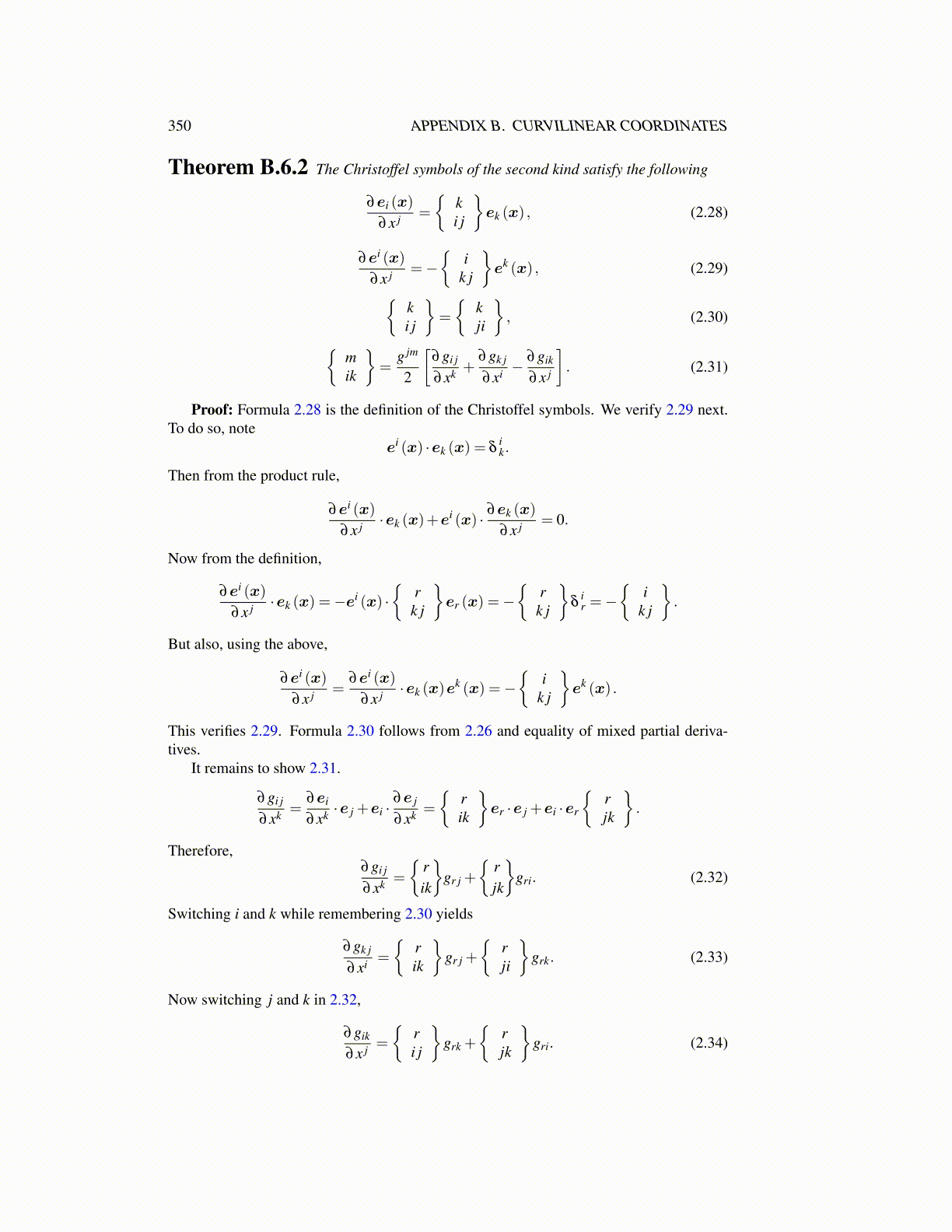
350 APPENDIX B. CURVILINEAR COORDINATES
Theorem B.6.2 The Christoffel symbols of the second kind satisfy the following
∂ei (x)
∂x j =
{ki j
}ek (x) , (2.28)
∂ei (x)
∂x j =−{
ik j
}ek (x) , (2.29){
ki j
}=
{kji
}, (2.30){
mik
}=
g jm
2
[∂gi j
∂xk +∂gk j
∂xi −∂gik
∂x j
]. (2.31)
Proof: Formula 2.28 is the definition of the Christoffel symbols. We verify 2.29 next.To do so, note
ei (x) ·ek (x) = δik.
Then from the product rule,
∂ei (x)
∂x j ·ek (x)+ei (x) · ∂ek (x)
∂x j = 0.
Now from the definition,
∂ei (x)
∂x j ·ek (x) =−ei (x) ·{
rk j
}er (x) =−
{r
k j
}δ
ir =−
{i
k j
}.
But also, using the above,
∂ei (x)
∂x j =∂ei (x)
∂x j ·ek (x)ek (x) =−
{i
k j
}ek (x) .
This verifies 2.29. Formula 2.30 follows from 2.26 and equality of mixed partial deriva-tives.
It remains to show 2.31.
∂gi j
∂xk =∂ei
∂xk ·e j +ei ·∂e j
∂xk =
{rik
}er ·e j +ei ·er
{rjk
}.
Therefore,∂gi j
∂xk =
{rik
}gr j +
{rjk
}gri. (2.32)
Switching i and k while remembering 2.30 yields
∂gk j
∂xi =
{rik
}gr j +
{rji
}grk. (2.33)
Now switching j and k in 2.32,
∂gik
∂x j =
{ri j
}grk +
{rjk
}gri. (2.34)