Chapter 13

Degree TheoryThis chapter is on the Brouwer degree, a very useful concept with numerous and importantapplications. The degree can be used to prove some difficult theorems in topology suchas the Brouwer fixed point theorem, the Jordan separation theorem, and the invariance ofdomain theorem. A couple of these big theorems have been presented earlier, but when youhave degree theory, they get much easier. Degree theory is also used in bifurcation theoryand many other areas in which it is an essential tool. The degree will be developed forRp inthis book. When this is understood, it is not too difficult to extend to versions of the degreewhich hold in Banach space. There is more on degree theory in the book by Deimling [11]and much of the presentation here follows this reference. Another more recent book whichis really good is [13]. This is a whole book on degree theory.

The original reference for the approach given here, based on analysis, is [24] and datesfrom 1959. The degree was developed earlier by Brouwer and others using different meth-ods. The more classical approach based on simplices and approximations with these is in[26]. I have given an approach based on singular homology as an appendix in [31].

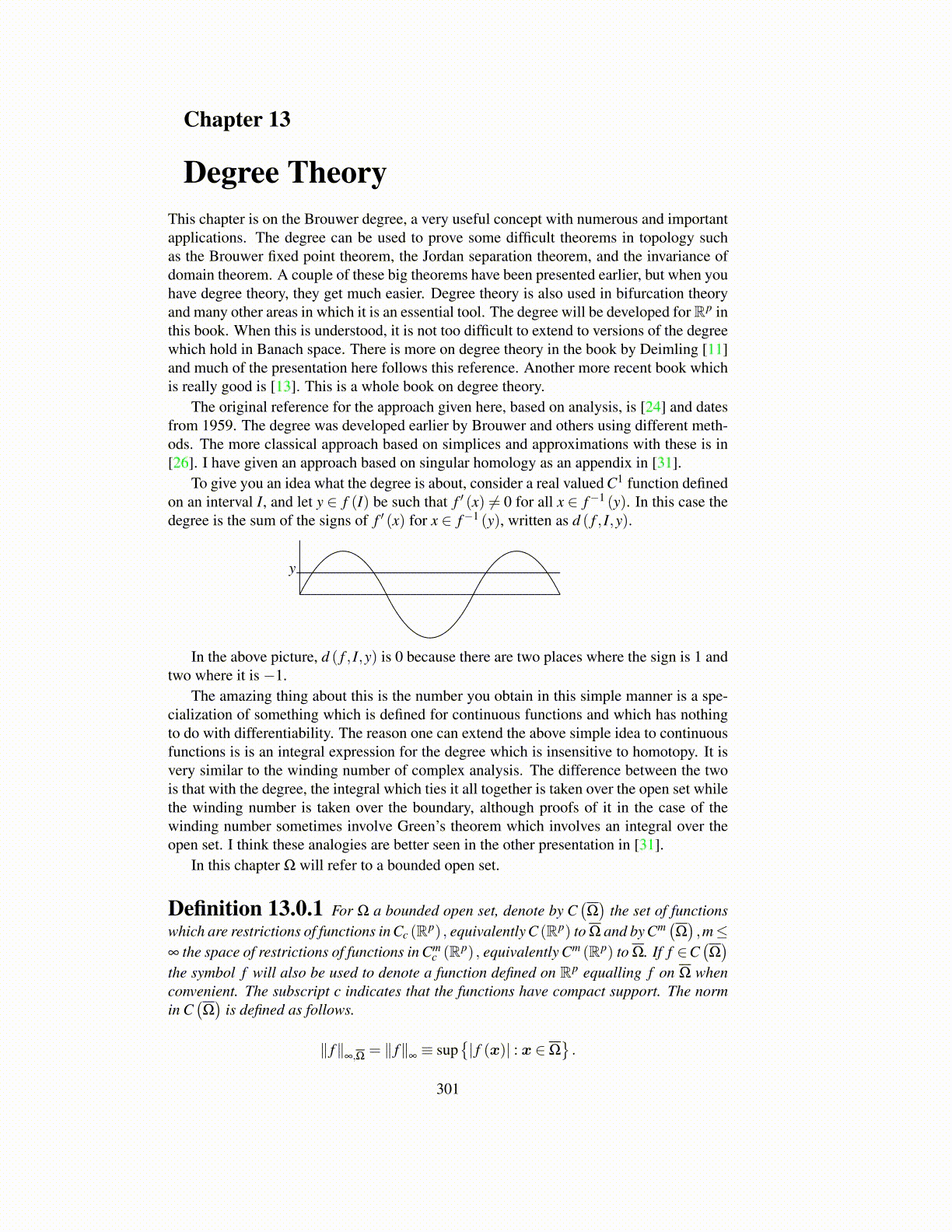
To give you an idea what the degree is about, consider a real valued C1 function definedon an interval I, and let y ∈ f (I) be such that f ′ (x) ̸= 0 for all x ∈ f−1 (y). In this case thedegree is the sum of the signs of f ′ (x) for x ∈ f−1 (y), written as d ( f , I,y).

y

In the above picture, d ( f , I,y) is 0 because there are two places where the sign is 1 andtwo where it is −1.

The amazing thing about this is the number you obtain in this simple manner is a spe-cialization of something which is defined for continuous functions and which has nothingto do with differentiability. The reason one can extend the above simple idea to continuousfunctions is is an integral expression for the degree which is insensitive to homotopy. It isvery similar to the winding number of complex analysis. The difference between the twois that with the degree, the integral which ties it all together is taken over the open set whilethe winding number is taken over the boundary, although proofs of it in the case of thewinding number sometimes involve Green’s theorem which involves an integral over theopen set. I think these analogies are better seen in the other presentation in [31].

In this chapter Ω will refer to a bounded open set.

Definition 13.0.1 For Ω a bounded open set, denote by C(Ω)

the set of functionswhich are restrictions of functions in Cc (Rp) , equivalently C (Rp) to Ω and by Cm

(Ω),m≤

∞ the space of restrictions of functions in Cmc (Rp) , equivalently Cm (Rp) to Ω. If f ∈C

(Ω)

the symbol f will also be used to denote a function defined on Rp equalling f on Ω whenconvenient. The subscript c indicates that the functions have compact support. The normin C

(Ω)

is defined as follows.

∥ f∥∞,Ω = ∥ f∥

∞≡ sup

{| f (x)| : x ∈Ω

}.

301