298 CHAPTER 12. THEOREMS INVOLVING LINE INTEGRALS
21. Show the Weierstrass approximation theorem will not work to approximate arbitrarycontinuous functions of a complex variable. Hint: You might use the Cauchy integralformula and the above problems to show that continuity is not enough.
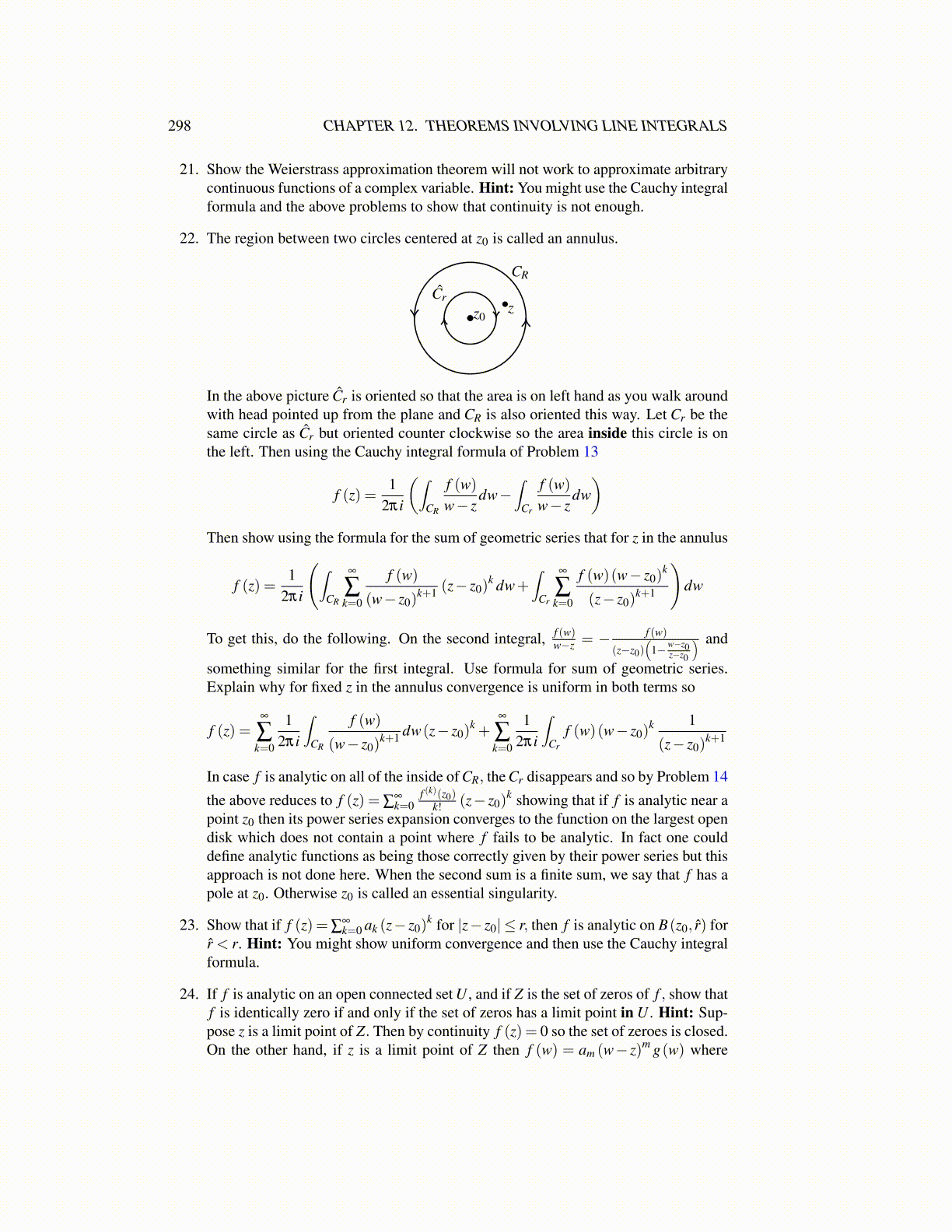
22. The region between two circles centered at z0 is called an annulus.
CR
Ĉrzz0
In the above picture Ĉr is oriented so that the area is on left hand as you walk aroundwith head pointed up from the plane and CR is also oriented this way. Let Cr be thesame circle as Ĉr but oriented counter clockwise so the area inside this circle is onthe left. Then using the Cauchy integral formula of Problem 13
f (z) =1
2πi
(∫CR
f (w)w− z
dw−∫
Cr
f (w)w− z
dw)
Then show using the formula for the sum of geometric series that for z in the annulus
f (z) =1
2πi
(∫CR
∞
∑k=0
f (w)
(w− z0)k+1 (z− z0)
k dw+∫
Cr
∞
∑k=0
f (w)(w− z0)k
(z− z0)k+1
)dw
To get this, do the following. On the second integral, f (w)w−z = − f (w)
(z−z0)(
1−w−z0z−z0
) and
something similar for the first integral. Use formula for sum of geometric series.Explain why for fixed z in the annulus convergence is uniform in both terms so
f (z) =∞
∑k=0
12πi
∫CR
f (w)
(w− z0)k+1 dw(z− z0)
k +∞
∑k=0
12πi
∫Cr
f (w)(w− z0)k 1
(z− z0)k+1
In case f is analytic on all of the inside of CR, the Cr disappears and so by Problem 14
the above reduces to f (z) = ∑∞k=0
f (k)(z0)k! (z− z0)
k showing that if f is analytic near apoint z0 then its power series expansion converges to the function on the largest opendisk which does not contain a point where f fails to be analytic. In fact one coulddefine analytic functions as being those correctly given by their power series but thisapproach is not done here. When the second sum is a finite sum, we say that f has apole at z0. Otherwise z0 is called an essential singularity.
23. Show that if f (z) = ∑∞k=0 ak (z− z0)
k for |z− z0| ≤ r, then f is analytic on B(z0, r̂) forr̂ < r. Hint: You might show uniform convergence and then use the Cauchy integralformula.
24. If f is analytic on an open connected set U , and if Z is the set of zeros of f , show thatf is identically zero if and only if the set of zeros has a limit point in U . Hint: Sup-pose z is a limit point of Z. Then by continuity f (z) = 0 so the set of zeroes is closed.On the other hand, if z is a limit point of Z then f (w) = am (w− z)m g(w) where