Chapter 12

Theorems Involving Line Integrals12.1 Green’s Theorem

Green’s theorem is an important theorem which relates line integrals to integrals over asurface in the plane. It can be used to establish the seemingly more general Stoke’s theorembut is interesting for it’s own sake. Historically, theorems like it were important in thedevelopment of complex analysis.

Here is a proof of Green’s theorem from the divergence theorem. This discussion isalso in “Calculus of One and Many Variables” which discusses line integrals or see Section11.5 for a short treatment based on the general case of differentiable manifolds.

Theorem 12.1.1 (Green’s Theorem) Let U be an open set in the plane for whichthe divergence theorem holds and let

F (x,y) = (P(x,y) ,Q(x,y))

be a C1 vector field defined near U. Then

∫∂U

F ·dR=∫

U

(∂Q∂x

(x,y)− ∂P∂y

(x,y))

dm2.

In the other notation, ∫∂U

Pdx+Qdy =∫

U(Qx−Py)dm2

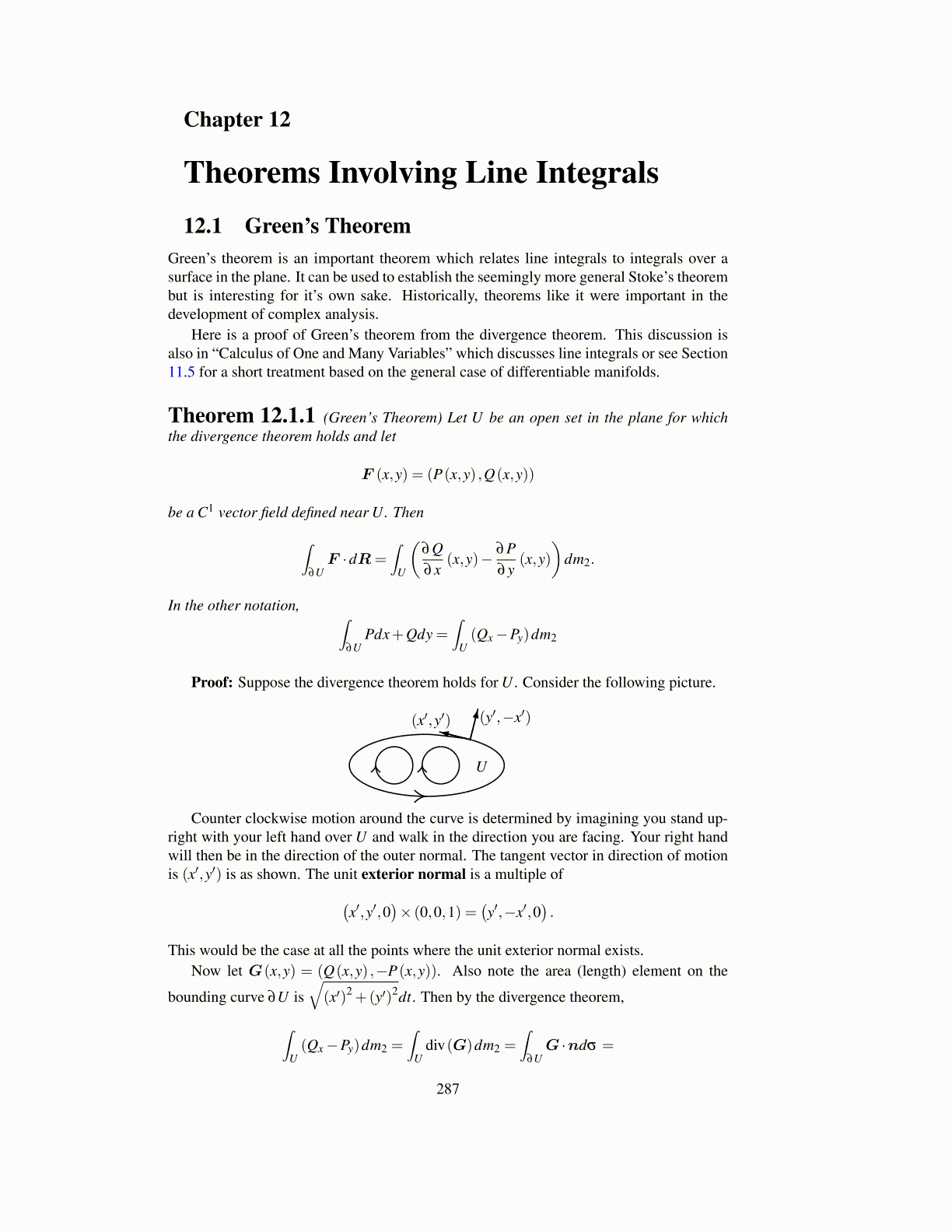
Proof: Suppose the divergence theorem holds for U . Consider the following picture.

(x′,y′) (y′,−x′)

U

Counter clockwise motion around the curve is determined by imagining you stand up-right with your left hand over U and walk in the direction you are facing. Your right handwill then be in the direction of the outer normal. The tangent vector in direction of motionis (x′,y′) is as shown. The unit exterior normal is a multiple of(

x′,y′,0)× (0,0,1) =

(y′,−x′,0

).

This would be the case at all the points where the unit exterior normal exists.Now let G(x,y) = (Q(x,y) ,−P(x,y)). Also note the area (length) element on the

bounding curve ∂U is√

(x′)2 +(y′)2dt. Then by the divergence theorem,

∫U(Qx−Py)dm2 =

∫U

div(G)dm2 =∫

∂UG ·ndσ =

287